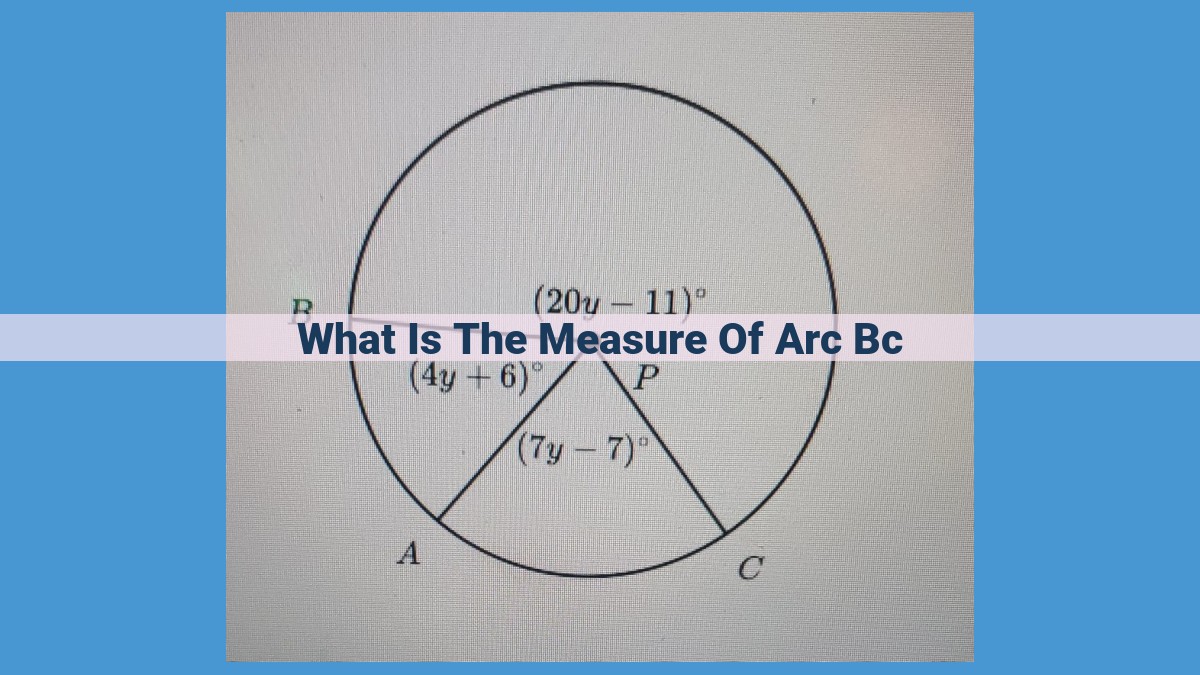
The measure of arc BC is determined by the measure of its corresponding central angle BOC. The formula to calculate the arc measure is: (measure of central angle BOC / 360) x 2πr, where r is the radius of the circle. Since the measure of central angle BOC and the radius are known, the measure of arc BC can be calculated using this formula, providing the length of the curved line segment on the circle.
Understanding the Measure of an Arc: A Geometrical Adventure
Step into the realm of geometry, where we embark on a captivating journey to unravel the mysteries of arcs and their enchanting connection with central angles.
Imagine yourself at the heart of a circle, a realm of perfect symmetry and boundless possibilities. Along its circumference lies an arc, a graceful crescent-shaped segment that adds a touch of curvature to this otherwise monotonous realm. But what truly sets an arc apart is its mystical bond with a central angle, an angle whose vertex resides at the circle’s center.
The measure of an arc, dear reader, holds a profound secret. It is intimately linked to the measure of its corresponding central angle. Just as the hands of a clock dance around its face, marking the passage of time, so too does the arc dance around the circle, its measure mirroring that of the central angle. This harmonious relationship, an embodiment of geometrical precision, reveals a captivating pattern.
To delve deeper into the enchantment of this geometrical affair, let us introduce Arc BC, a specific arc gracing our circular canvas. Arc BC, like all arcs, possesses a unique identity, a numerical value that quantifies its extent. This value, dear reader, is directly proportional to the measure of its central angle, BOC. The wider the central angle BOC spreads its wings, the greater the measure of Arc BC.
But the tale of Arc BC and its central angle doesn’t end there. These two geometrical entities are bound together by a formula, an equation that encapsulates their inseparable bond. This formula, a beacon of geometrical wisdom, allows us to determine the measure of Arc BC with ease, knowing only the measure of central angle BOC and the circle’s radius.
So, let us unravel the enigma of the arc’s measure, a measure that reflects the grace of geometry. As we journey through this mathematical landscape, may you discover the captivating harmony that exists between arcs and central angles, a harmony that paints the world of geometry in vibrant hues.
Defining the Central Angle
Understanding Central Angles
In the realm of circles, understanding central angles is crucial for mastering the geometry of these perfect shapes. A central angle is an angle formed by two radii that intersect at the center of a circle. It serves as the gatekeeper between the arc it encompasses and the heart of the circle.
The Power of Radii
Radii, defined as line segments connecting the center to any point on the circle, play a pivotal role in forming central angles. They act as the gatekeepers, determining the span of the angle at the center. Imagine two radii gracefully dancing around the center, their intersection creating two majestic arcs.
Center Stage
The center of a circle, like a silent conductor, orchestrates the harmony of radii and angles. The central angle’s reach is defined by its position relative to this central point. By aligning the starting point of two radii at the center, we define the base of the central angle.
Arc BC and Its Properties
As we explore the world of arcs and central angles, let’s focus on a specific arc, denoted as Arc BC. An arc, like a curved smile, is a portion of a circle’s circumference. Arc BC resides on the circumference of our circle, connecting two points, B and C.
Central Angle BOC
Every arc is closely intertwined with a central angle, much like a shadow following a person. The central angle BOC is formed by two radii of our circle, OA and OC, which intersect at the circle’s heart, the center. This central angle, like a beacon, shines its light on the arc, dictating its size.
Proportionality and Measurement
A fascinating relationship exists between Arc BC and central angle BOC: they are directly proportional. Just as two dancers move in sync, the measure of Arc BC and the measure of central angle BOC dance together in harmony. If the angle increases, so does the arc’s length, and vice versa.
Formula for Arc Measure
To determine the measure of Arc BC, we have a trusty formula at our disposal:
Measure of Arc BC = (Measure of Central Angle BOC / 360) × 2πr
where r represents the radius of the circle.
This formula serves as a compass, guiding us to calculate the arc’s length based on the central angle and the circle’s radius. It’s like deciphering a secret code, revealing the mysteries of geometry.
Example
Say we have a circle with a radius of 5 units and central angle BOC measures 60 degrees. Plugging these values into our formula:
Measure of Arc BC = (60 / 360) × 2π(5) ≈ 5.24 radians
Arc BC and central angle BOC are inseparable companions, embarking on a journey of exploration together. Their direct proportionality and the formula for arc measure empower us to unravel the secrets of circles and the fascinating world of geometry.