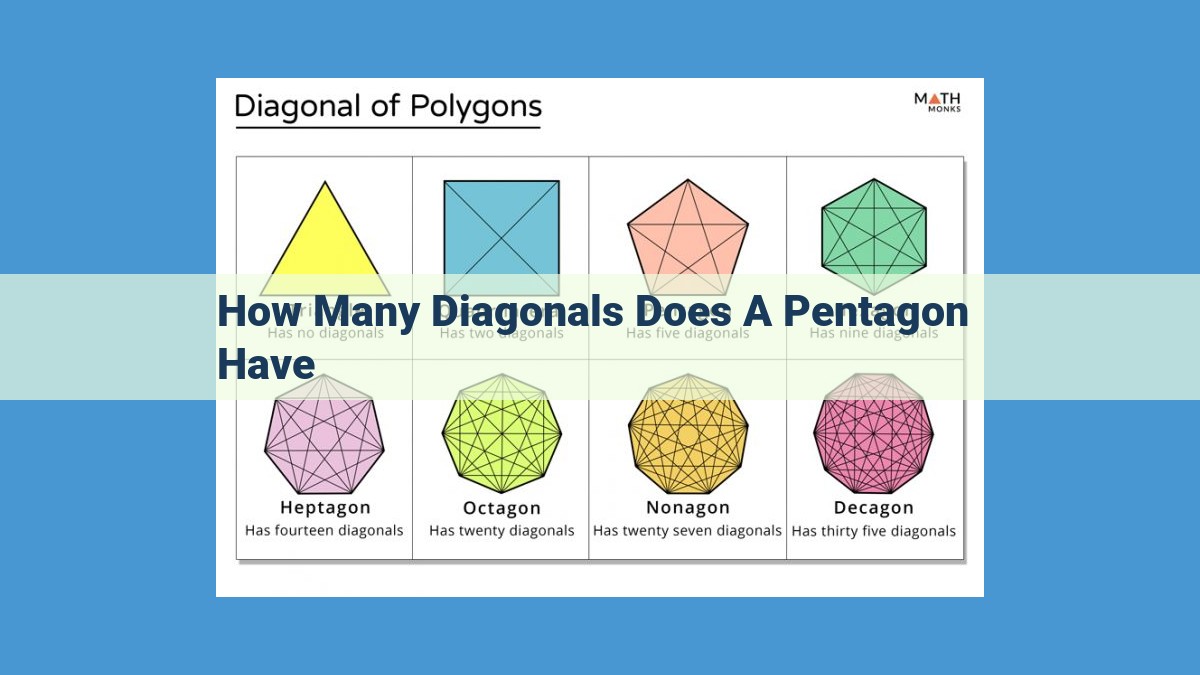
A pentagon, a polygon with five sides, has diagonals that connect non-consecutive vertices. To calculate the number of diagonals (d) in a polygon with n sides, the formula d = n(n-3)/2 is used. Applying this formula to a pentagon (n = 5), we get d = 5(5-3)/2 = 5(2)/2 = 5. Therefore, a pentagon has 5 diagonals. Understanding geometry and the properties of polygons is crucial for solving such problems.
Let’s embark on a geometric journey, starting with a fascinating shape: the polygon. A polygon is a closed figure formed by straight lines, like a puzzle piece with multiple sides. These special shapes boast unique characteristics, such as their number of sides, angles, and diagonals.
Geometry, the study of shapes and their properties, invites us to delve into the intriguing world of polygons. It’s a language of shapes, angles, and patterns that helps us understand the geometric tapestry of our surroundings. From the hexagonal honeycomb to the pentagonal soccer ball, polygons shape our world in countless ways.
Understanding the Pentagon: The Enigmatic Polygon with Five Sides
In the vast realm of geometry, where shapes dance and patterns unfold, the pentagon emerges as an enigmatic figure. Defined by its five sides and captivating symmetry, the pentagon has captured the imagination of mathematicians and architects alike.
Polygonal Tapestry and Geometrical Exploration
Polygons, a diverse family of shapes, are defined by their straight sides and closed boundaries. The number of sides plays a crucial role in classifying polygons. From triangles with three sides to hexagons with six sides, each polygon possesses unique characteristics and properties.
Our focus, however, is on the pentagon, a mysterious figure with five sides. Its name, derived from the Greek words “penta” (five) and “gon” (angle), reveals its unique identity. Intriguingly, the pentagon holds a special place in geometry, as it is the first regular polygon with an odd number of sides.
Understanding Diagonals in Polygons: Unveiling the Secrets of Shape Geometry
Understanding the intricacies of polygonal geometry is a fundamental aspect of mathematical exploration. In this blog post, we’ll delve into the fascinating world of diagonals and explore their significance in polygons, particularly the pentagon.
What’s a Diagonal?
A diagonal is a line segment that connects two non-consecutive vertices of a polygon. In other words, it’s a line that cuts across the polygon, not along its sides.
Formula for Diagonals in Polygons
Mathematicians have devised a clever formula that allows us to calculate the number of diagonals in a polygon with n sides:
Diagonals = n(n-3)/2
This formula is a powerful tool that simplifies the process of counting diagonals.
Diagonals in a Pentagon
Applying the diagonal formula to a pentagon, where n equals 5, we get:
Diagonals = 5(5-3)/2 = 5(2)/2 = 5
Therefore, a pentagon has 5 diagonals.
Understanding geometry is essential for comprehending the world’s shapes and structures. By delving into the concept of diagonals, we’ve gained valuable insights into the properties of polygons, particularly the pentagon. This knowledge empowers us to solve complex geometry problems and appreciate the beauty and order that mathematics brings to our understanding of the world.
Unlocking the Secrets of Polygons: Diagonals in a Pentagon
Our journey begins with the intriguing realm of polygons, the fundamental building blocks of geometry. Polygons, as their name suggests, are closed figures formed by straight line segments that meet at points called vertices. One such polygon that holds a special place in our exploration is the pentagon, a shape with five sides and five vertices.
Understanding the Pentagon: A Unique Shape
A pentagon is a polygon with five sides. Polygons are further classified based on the number of their sides, with the pentagon belonging to the class of pentagrams.
Diagonals in Polygons: A Concept Revealed
Now, let’s venture into a slightly more complex concept: diagonals. Diagonals are straight line segments that connect two non-consecutive vertices of a polygon. Every polygon has a certain number of diagonals, and we can determine this number using a handy formula:
Diagonals = n(n-3)/2
where n represents the number of sides of the polygon.
Counting Diagonals in a Pentagon: A Practical Application
Applying this formula to our pentagon, we get:
Diagonals = 5(5-3)/2
Diagonals = 5(2)/2
Diagonals = 5
So, a pentagon has 5 diagonals. This result highlights the power of geometry in solving problems related to shapes and their properties. By understanding the concept of diagonals and using the appropriate formula, we can effortlessly determine the number of diagonals in a pentagon.
Embracing the Geometry of Our World
Geometry is not just an academic subject confined to textbooks; it’s a tool that helps us make sense of the world around us. From the patterns in nature to the structures we build, geometry plays a vital role in our daily lives. Understanding geometry empowers us to appreciate the beauty and functionality of the shapes that surround us.