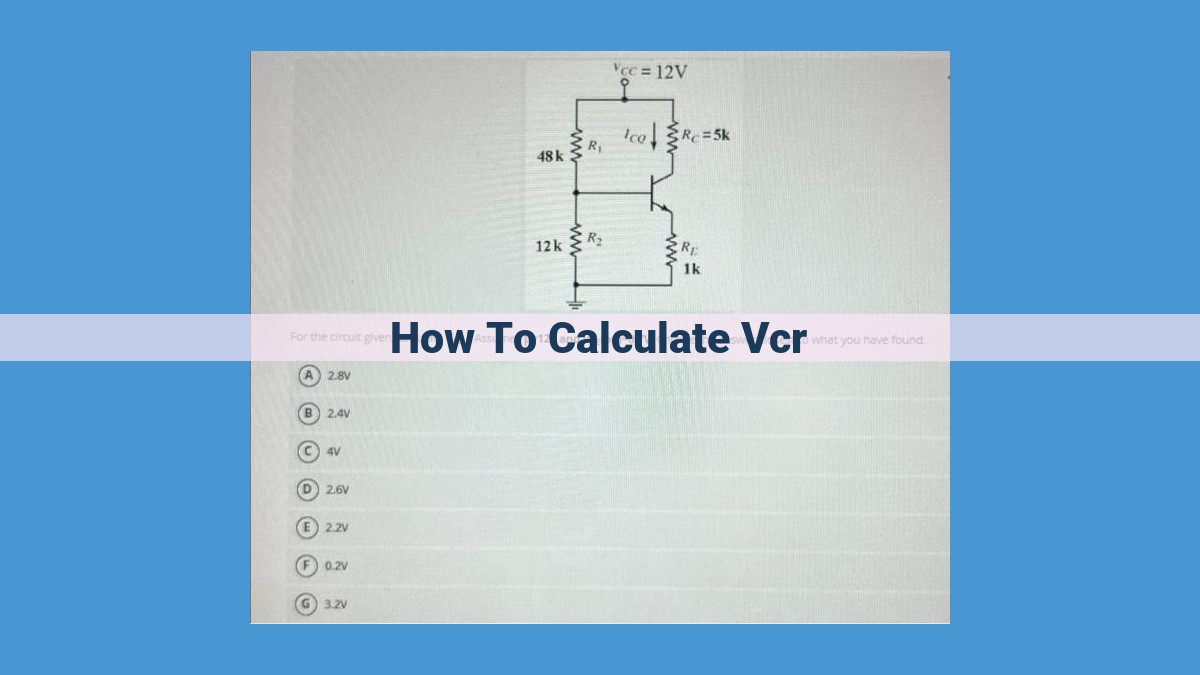
To calculate VCR (Variable cost ratio), gather data on variable costs and sales revenue for a specific period. Calculate variable costs as a percentage of sales revenue by dividing total variable costs by total sales revenue. Express the result as a decimal or a percentage. For example, if variable costs amount to $50,000 and sales revenue is $100,000, the VCR is 50% or 0.5.
Understanding the Rate of Return:
- Define rate of return and provide its formula.
- Explain its relationship with present value, future value, and annuities.
- Discuss the time value of money principles.
Understanding the Rate of Return: A Journey through Time Value
In the world of finance, the rate of return plays a pivotal role in deciphering the relationship between time and money. It measures the percentage rate at which an investment earns profits over a given period, providing insights into the growth potential of financial assets.
Mathematically, the rate of return can be expressed as:
Rate of Return = (Future Value - Present Value) / Present Value
This equation unravels the interplay between three crucial concepts:
-
Present Value (PV): The current worth of a future sum of money, discounted at a rate of return.
-
Future Value (FV): The estimated worth of a present sum of money in the future, assuming a specific rate of return.
-
Annuities: A series of periodic payments, received or paid over a set time, with each payment representing a constant sum.
These concepts intertwine with the time value of money principle, which emphasizes that the value of money varies over time due to its potential earning capacity. A dollar today is worth more than a dollar in the future, as it has the ability to generate additional income through investments.
By understanding the rate of return and its intricate connections with present value, future value, and annuities, you gain the power to make informed financial decisions. It empowers you to calculate the potential earnings of investments, estimate future financial obligations, and plan for long-term financial stability.
Annuities: The Power of Recurring Cash Flows
In the realm of personal finance, annuities stand as a cornerstone, offering a guaranteed source of income that can make a significant impact on your financial future. Whether you’re planning for retirement, saving for a special purchase, or simply seeking a stable financial foundation, annuities provide a unique and valuable tool.
What is an Annuity?
An annuity is a contract between you and an insurance company where you make a lump sum payment or a series of regular deposits. In return, the insurance company guarantees to pay you a series of regular income payments over a specific period or even for the rest of your life.
Types of Annuities
There are two main types of annuities:
- Ordinary (Deferred) Annuities: With this type, you make a lump sum payment or a series of deposits, and the insurance company starts making income payments at a later agreed-upon date, such as retirement.
- Due (Immediate) Annuities: With this type, you make a lump sum payment, and the insurance company immediately starts making income payments to you.
Calculating the Present and Future Value of Annuities
Understanding the present and future value of an annuity is crucial for making informed financial decisions. The present value represents the current value of all future income payments, while the future value represents the total value of the annuity at a future date, taking into account the rate of return.
The Interplay with Rate of Return and Time Value of Money
The rate of return and time value of money play a significant role in determining the value of an annuity. A higher rate of return will result in a higher future value, while a longer time period will result in a lower present value.
Applications
Annuities have a wide range of applications, including:
- Retirement planning
- Saving for a child’s education
- Generating a steady stream of income
- Establishing a legacy for beneficiaries
Annuities offer a powerful and versatile way to secure your financial future. By understanding the concepts of present and future value, as well as the interplay with rate of return and time value of money, you can make informed decisions about using annuities to achieve your financial goals. Whether you’re seeking a steady income stream or planning for a major purchase, annuities provide a flexible and guaranteed way to grow your wealth and ensure a brighter financial future.
Present Value: Discounting Future Cash Flows
In the world of finance, the concept of present value plays a crucial role in determining the worth of future cash flows. It’s like a magic wand that allows us to bring future money back to the present and compare it with today’s value.
So, what exactly is this discounting business all about? Well, it’s a way of accounting for the time value of money. Picture this: A dollar today is worth more than a dollar tomorrow because you could invest it and earn interest over time. That’s why we need to adjust future cash flows to reflect their present worth.
How Does Discounting Work?
To calculate the present value of a future cash flow, we use a discount rate. This rate represents the return you expect to earn on an investment over the same period. The formula for present value is:
Present Value = Future Value / (1 + Discount Rate)^Number of Periods
Let’s say you’re expecting a payment of $1,000 in 5 years, and the prevailing interest rate is 5%. Using the formula, we can calculate the present value as:
Present Value = 1,000 / (1 + 0.05)^5 = $783.53
This means that the $1,000 you’ll receive in 5 years is worth only $783.53 today, given the 5% discount rate.
Relationships with Other Concepts
Present value is closely linked to other financial concepts:
- Rate of return: The discount rate used in present value calculations is typically the rate of return you could earn on an alternative investment.
- Future value: Present value is the inverse of future value. You can use the same formula to calculate future value, but with the discount rate flipped to the power of the number of periods.
- Annuities: Annuities are a series of regular payments or receipts. Present value can be used to determine the value of an annuity’s future cash flows, providing insights into investment or retirement planning.
Practical Applications
Present value calculations have wide-ranging applications in finance, including:
- Investment valuation: Determining the fair value of investments based on their expected future cash flows.
- Financial planning: Planning for retirement or other long-term financial goals by calculating the present value of future income and expenses.
- Loan repayments: Calculating the loan amount needed to finance a purchase based on the present value of future repayments.
By understanding the concept of present value, you empower yourself to make informed financial decisions. It’s like having a time machine for your money, allowing you to compare future cash flows with today’s value, and plan for a more secure financial future.
Future Value: Compounding Future Cash Flows
In the realm of personal finance and investing, understanding the concept of future value is paramount. Future value refers to the amount an investment or loan will be worth at a specific point in the future, taking into account the power of compounding.
Compounding is the snowball effect of earning interest on both your initial investment and the accumulated interest from previous periods. Over time, this exponential growth can significantly magnify your returns.
The relationship between future value, present value (the initial investment), rate of return, and time is intricately intertwined. The higher the rate of return and the longer the investment period, the greater the future value.
Let’s consider an example: If you invest $1,000 today at a 5% annual rate of return, in 10 years, your investment will grow to over $1,628. This is because the interest earned in the first year ($50) is added to your original investment, and in the second year, you earn interest not only on the initial $1,000 but also on the accumulated $50. This effect continues year after year, resulting in a larger and larger future value.
This concept is also crucial in loan repayments. For example, if you borrow $10,000 at a 4% annual interest rate for 10 years, you will repay a total of $14,693. This includes the initial $10,000 loan amount plus the accumulated interest of $4,693.
Understanding future value is essential for making informed financial decisions. By considering the impact of compounding, you can optimize your investment strategy, plan for future expenses, and manage your debt effectively. Embrace the power of future value and unlock the potential for substantial financial growth over time.
Time Value of Money: The Cornerstone of Financial Decision-Making
In the realm of personal finance and investing, “Time” plays a pivotal role. The concept of Time Value of Money (TVM) is a fundamental principle that helps us understand the impact of time on the value of money.
Defining Time Value of Money
Simply put, TVM recognizes that the value of money today is not the same as its value in the future. Today’s dollar is worth more than tomorrow’s dollar. This is because money invested today has the potential to grow through interest or returns, while money held in the future has already missed out on those opportunities.
Connecting Rate of Return, Present Value, and Future Value
TVM establishes a crucial link between the rate of return, present value, and future value of money. The rate of return reflects the percentage growth of money over time. Present value calculates the current worth of a future sum of money, discounted by the rate of return. Conversely, future value determines the value of a present sum of money at a future date, taking into account the rate of return.
Applications in Financial Decision-Making
The significance of TVM cannot be understated in the realm of financial decision-making. It empowers us to:
- Compare investment options: By calculating the present value of future cash flows, we can determine which investment offers the highest return for a given level of risk.
- Plan for retirement: TVM helps us estimate the future value of our retirement savings, ensuring we accumulate enough to maintain our desired lifestyle.
- Evaluate financing options: The present value of future loan payments can reveal which loan option presents the most cost-effective solution.
A Story to Illustrate TVM
Imagine you’re saving for a down payment on a house. You have $20,000 to invest today and the bank offers a 5% annual interest rate.
Using TVM, you can determine how much your investment will grow over time. In 5 years, your investment will have a future value of $26,373. This is because the interest earned on your initial investment will be compounded annually, increasing the total value over time.
In contrast, if you waited 5 years to begin saving, you would need to invest $29,904 at the same 5% interest rate to accumulate the same $26,373. This demonstrates the time value of money and the importance of starting to save and invest as early as possible.
Compounding and Discounting: Power of Time:
- Provide formulas and explanations for compounding (future value) and discounting (present value).
- Demonstrate practical applications of these concepts.
- Discuss their role in understanding time-related effects on financial values.
Compounding and Discounting: Harnessing the Power of Time
In the world of finance, time plays a pivotal role in shaping the value of money. Two fundamental concepts that capture this relationship are compounding and discounting, which are essential tools for understanding how money grows or diminishes over time.
Compounding: The Exponential Growth of Future Value
Compounding is the process by which future value increases at an exponential rate. This occurs when interest or earnings are added to the principal, and then interest is earned on the accumulated sum in subsequent periods. The formula for compounding is:
Future Value = Present Value * (1 + Rate of Return)^Number of Periods
For example, if you invest $100 at a 5% annual interest rate for 10 years, the future value will be:
Future Value = $100 * (1 + 0.05)^10 = $162.89
Discounting: The Inverse of Compounding
Discounting is the inverse of compounding, where the present value of a future sum of money is calculated. This is useful for determining the current worth of a future cash flow. The formula for discounting is:
Present Value = Future Value / (1 + Rate of Return)^Number of Periods
Using the same example as before, if you know that you will receive $162.89 in 10 years, and the current interest rate is 5%, the present value of that future amount is:
Present Value = $162.89 / (1 + 0.05)^10 = $100
Practical Applications of Compounding and Discounting
These concepts have numerous practical applications in finance, including:
- Calculating investment returns: Compounding is used to determine how much an investment will grow over time.
- Paying off loans: Discounting is used to calculate the present value of future loan payments, helping you understand the total cost of borrowing.
- Understanding annuities: Annuities are a series of periodic payments. Compounding and discounting are used to calculate the present value and future value of annuity payments.
By understanding compounding and discounting, you can make informed financial decisions that take into account the time value of money. These powerful concepts give you the ability to project the growth of your investments, plan for future expenses, and make the most of your financial resources.
Applications of VCR Concepts in Real-World Finance
The concepts of value, capital, and return (VCR) are fundamental pillars of financial decision-making. They play a crucial role in various financial scenarios, from investing to borrowing to planning for the future.
Calculating Investment Returns
When you invest, you expect a return on your investment. VCR concepts can help you calculate that return. The future value of your investment represents the expected value of your investment at a future date, taking into account the rate of return and the time period. By comparing the present value of your investment to its future value, you can determine its rate of return.
Example: Let’s say you invest $1,000 at a 5% annual rate of return for 10 years. Using the future value formula, you can calculate that your investment will be worth $1,628.89. This represents a rate of return of 5% per year.
Estimating Loan Payments
If you’re taking out a loan, VCR concepts can help you estimate your monthly payments. The present value of your loan is the total amount you owe, and the rate of return is the interest rate on the loan. By using the annuity formula, you can calculate the equal monthly payments that will satisfy the loan over a specified period.
Example: Let’s say you take out a $20,000 loan at a 3% annual interest rate for 5 years. The monthly payment, calculated using the annuity formula, would be $365.54.
Planning for the Future
VCR concepts are essential for planning your financial future. By understanding the time value of money, you can calculate how much you need to save today to reach a specific financial goal in the future. The present value of your desired future value represents the amount you need to invest today, taking into account the rate of return and the time period.
Example: Let’s say you want to have $100,000 in your retirement account in 20 years. Assuming an 8% annual rate of return, the present value of your goal is $24,461.11. By investing this amount today, you can reach your financial goal in the future.
Mastering VCR concepts empowers you to make informed financial decisions that can help you reach your financial goals, manage your finances effectively, and plan for a secure financial future.