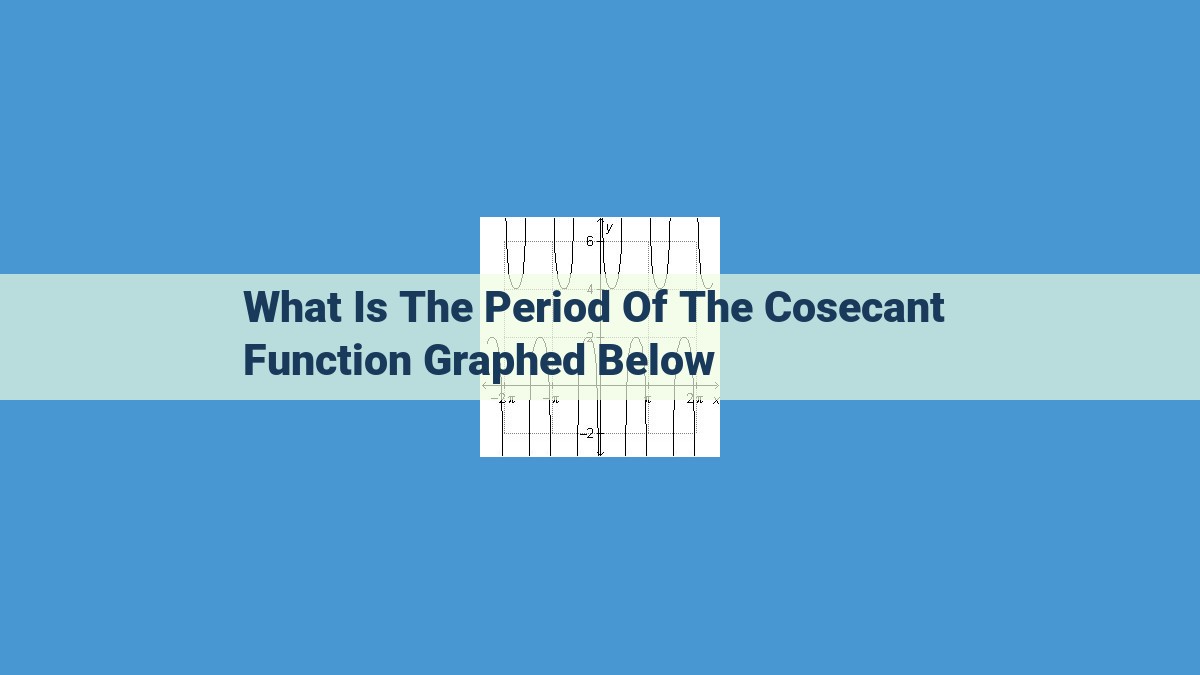
The period of the cosecant function, defined as the horizontal distance between successive maxima or minima, is 2π. This can be derived from the periods of its reciprocal functions, sine and cosine, which have periods of 2π and π, respectively. The period of cosecant is directly related to the period of sine, with the period of cosecant being twice the period of sine. Understanding the period of the cosecant function is crucial for analyzing and graphing trigonometric functions, as it helps determine the function’s behavior and key features.
- Define the cosecant function and its relationship to sine and cosine.
- Explain the concept of period in trigonometric functions.
Unlocking the Secrets of the Cosecant Function: A Journey Through Periodicity
In the realm of mathematics, trigonometric functions hold a special place, describing the cyclical patterns found in countless real-world phenomena. Among these functions, the cosecant stands out as a fascinating and essential tool. It’s a function that’s intimately connected to sine and cosine, the two fundamental trigonometric functions.
The cosecant function, denoted as csc(x), is defined as the reciprocal of the sine function: csc(x) = 1/sin(x). This unique relationship between csc(x), sin(x), and cosine (cos(x)) gives rise to intriguing properties, including its distinctive period.
Periodicity: A Fundamental Property of Trigonometric Functions
Periodicity is a defining characteristic of trigonometric functions. It refers to the regular, repetitive pattern that these functions exhibit as their input values vary. The period of a trigonometric function represents the horizontal distance between two consecutive peaks or troughs.
Determining the Period of the Cosecant Function
To understand the period of the cosecant function, we must first consider the periods of sine and cosine. The sine function has a period of 2π, meaning that its graph repeats itself every 2π units along the horizontal axis. Similarly, the cosine function has a period of 2π.
Since csc(x) is defined as 1/sin(x), its period is inversely related to the period of sine. Therefore, the period of csc(x) is:
Period of csc(x) = 2π/|period of sin(x)|
Example: Graphing and Calculating the Period
Let’s consider the function csc(x). Its graph will exhibit the characteristic repeating pattern of the cosecant function. To determine its period, we first identify the period of sine, which is 2π.
Plugging this value into the formula, we get:
Period of csc(x) = 2π/2π = 1
This means that the graph of csc(x) repeats itself every 1 unit along the horizontal axis, confirming the inverse relationship between the periods of csc(x) and sin(x).
Period of the Cosecant Function
Understanding the Rhythm of Trigonometric Functions
In the world of mathematics, trigonometric functions play a pivotal role in describing the rhythmic patterns we observe in fields like physics, engineering, and music. To fully grasp these patterns, one key concept we must explore is the period, which represents the horizontal distance between successive repetitions of a function’s cycle.
Defining the Cosecant Function
The cosecant function, denoted as cosec(x), is defined as the reciprocal of the sine function:
cosec(x) = 1/sin(x)
This function shares a fundamental relationship with its trigonometric siblings, the sine and cosine functions.
Period of a Trigonometric Function
The period of a trigonometric function is the distance it travels along the horizontal axis to complete one full cycle. For instance, the sine and cosine functions have a period of 2π.
Deriving the Period of Cosecant
The period of the cosecant function can be derived from the periods of sine and cosine. Since cosec(x) = 1/sin(x), its period must be the same as the period of its denominator, sine. Therefore, the period of cosecant is:
Period of cosec(x) = Period of sin(x) = 2π
Relating to Sine
The period of cosecant is directly related to the period of sine. Since the period of sine is 2π, the period of cosecant is also 2π. This means that the cosecant function repeats its values every 2π units along the horizontal axis.
Understanding the period of trigonometric functions is crucial for analyzing their behavior and graphing them accurately. The cosecant function, with its period of 2π, aligns precisely with the periods of sine and cosine. This relationship highlights the interconnected nature of trigonometric functions and their rhythmic patterns that underpin the world around us.
The Period of the Cosecant Function: Unveiling the Rhythm of Trigonometric Identity
In the realm of trigonometry, functions like sine, cosine, and their reciprocal counterparts play a fundamental role in understanding the periodic nature of the natural world. Among these, the cosecant function, represented by cosec(x), stands out as the reciprocal of the sine function. This profound relationship gives rise to an intriguing question: what is the period of the cosecant function?
The period of a trigonometric function refers to the horizontal distance between successive maxima or minima on its graph. In the case of sine and cosine, their periods are determined by the underlying unit circle. For sine, the period is 2π, while for cosine, it is also 2π.
Unveiling the Cosecant’s Period
The period of the cosecant function is directly linked to the periods of sine and cosine. Since cosec(x) = 1/sin(x), the period of cosecant is inversely related to that of sine. This means that the period of the cosecant function is π, half the period of sine.
In other words, over an interval of π, the cosecant function completes one full cycle, returning to its initial value. This shorter period compared to sine and cosine stems from the fact that the cosecant function oscillates more rapidly, alternating between positive and negative values.
Digging Deeper into Related Concepts
Period of Sine and Cosine:
The period of the sine function is 2π, representing the horizontal distance between consecutive maxima or minima along its graph. Similarly, the period of the cosine function is also 2π.
Amplitude of Sine and Cosine:
The amplitude of a trigonometric function refers to the vertical distance between its maximum and minimum values. For sine and cosine, the amplitude is 1. This indicates that their graphs oscillate between positive and negative values, with a maximum value of 1 and a minimum value of -1.
Understanding the Period’s Significance
Comprehending the period of trigonometric functions is crucial for analyzing and graphing these functions. The period determines the repeating pattern of the function’s oscillations, allowing us to accurately predict its behavior over any interval. It serves as a foundation for understanding how trigonometric functions model periodic phenomena in various real-world applications.
The period of the cosecant function is π, reflecting its reciprocal relationship with the sine function. This shorter period results in more frequent oscillations between positive and negative values. Together with the period and amplitude of sine and cosine, the cosecant’s period forms an essential framework for understanding and utilizing trigonometric functions in diverse scientific and mathematical domains.
Unveiling the Period of the Elusive Cosecant Function
Trigonometric functions, such as sine, cosine, and their intriguing relative, the cosecant function, possess a fundamental characteristic known as period. Understanding this elusive concept is pivotal for analyzing and graphing these functions with precision.
Period in Trigonometric Functions
The period of a trigonometric function, like a silent yet steady heartbeat, represents the horizontal distance between consecutive identical points, be it a maximum, minimum, or zero-crossing. This rhythmic repetition defines the function’s fundamental pattern.
Period of the Cosecant Function
The cosecant function, the reciprocal of sine, inherits its period from its sineful counterpart. The period of cosecant equals the period of sine.
Relationship to Sine and Cosine
The divine connection between sine and cosine extends to their periods. The period of sine, denoted by 2π, reflects the horizontal distance between successive maxima or minima. Cosine, its mirror image across the x-axis, shares this period, signifying the distance between consecutive maxima or minima.
Example
Consider the cosecant function csc(x). Its graph, like a graceful dancer, repeats its sinusoidal pattern every 2π units along the x-axis. Each crest and trough, marking a maximum and minimum, respectively, occurs precisely 2π units apart.
Comparing Periods
The period of cosecant, inextricably linked to sine, stands in stark contrast to the period of cosine. While both sine and cosine possess a period of 2π, cosecant’s period is twice that of cosine. This duality stems from the reciprocal relationship between cosecant and sine.
The period of the cosecant function, entwined with the rhythm of sine and cosine, provides a vital compass for navigating the ever-changing landscape of trigonometry. Understanding this concept empowers us to analyze, graph, and comprehend the intricate dance of trigonometric functions with ease.