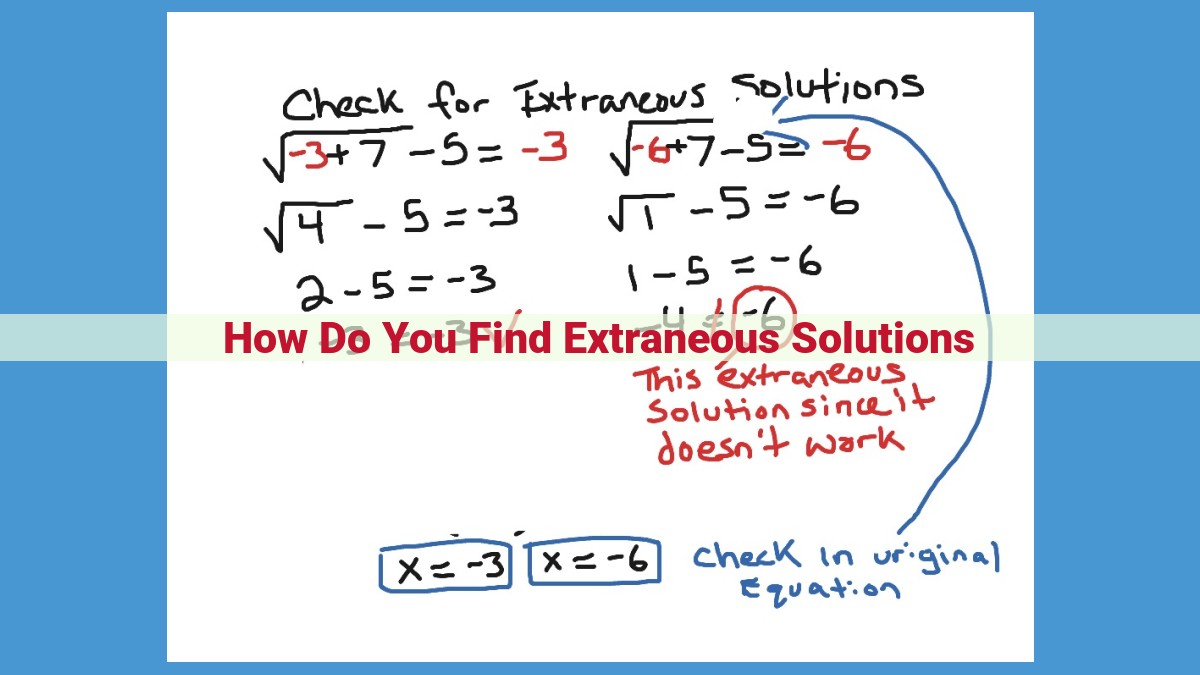
To find extraneous solutions, first substitute potential solutions back into the original equation to verify they satisfy it. Additionally, check if solutions fall within the domain using interval notation, or graph the equation to visualize the solutions and identify any points that do not satisfy the constraints given. By examining potential solutions using these methods, extraneous solutions can be identified and eliminated, ensuring the accuracy of the solution set.
Understanding Extraneous Solutions: A Guide to Accurate Equation Solving
In the realm of mathematics, equations and inequalities reign supreme, providing us with tools to unravel relationships between variables. However, these equations can sometimes yield deceptive solutions known as extraneous solutions, which take us down the wrong path. Identifying and eliminating extraneous solutions is crucial for finding the true solutions that accurately solve our mathematical dilemmas.
Extraneous solutions arise when we encounter equations or inequalities that get simplified during the solution process, potentially introducing new solutions that are not valid within the original context. These solutions may seem plausible at first, but they often hide pitfalls that can lead to incorrect conclusions. To prevent such pitfalls, it is essential to scrutinize our solutions and check for any hidden constraints.
In the following sections, we will delve into the various methods for identifying and eliminating extraneous solutions. Equipping ourselves with these techniques will empower us to tackle equations and inequalities with confidence, knowing that we are finding the most accurate solutions possible.
Methods for Finding Extraneous Solutions
When solving equations and inequalities, you may encounter solutions that don’t make sense in the original context. These are called extraneous solutions. It’s crucial to find and eliminate them to ensure accurate results. Here are three effective methods to identify and discard extraneous solutions:
Substitution
The simplest method is substitution. After you find a solution, plug it back into the original equation or inequality. If the result is not true, then the solution is extraneous. For example, consider the equation x^2 – 4 = 0. The solutions are x = 2 and x = -2. Substituting x = 2, we get 2^2 – 4 = 0, which is true. But when we substitute x = -2, we get (-2)^2 – 4 = 0, which is not true. Therefore, x = -2 is an extraneous solution.
Interval Notation
Interval notation is a handy tool when the equation or inequality has a restricted domain. It involves checking if the solutions lie within the domain. For instance, solving the inequality 2x + 1 > 5, where x ≥ 0, would yield x > 2. However, since the domain is restricted to non-negative values, the solution must be x ≥ 2. This eliminates any solutions less than 2.
Graphing
Graphing the equation or inequality can provide a visual representation of the solutions. By plotting points and examining the graph, you can identify any solutions that don’t make sense. For example, graphing the equation y = x^2 – 1 shows that the graph opens upwards, indicating that all solutions are non-negative. Therefore, if you find a solution that is negative, it would be extraneous.
Checking for Extraneous Solutions: A Step-by-Step Guide
When solving equations or inequalities, it’s crucial to check for extraneous solutions – solutions that satisfy the simplified equation but not the original equation or inequality. These seemingly valid solutions can lead to incorrect conclusions and hinder your understanding. Let’s delve into a step-by-step approach to identify and eliminate extraneous solutions.
Step 1: Recall the Methods for Finding Extraneous Solutions
- Substitution: Plug the solution back into the original equation or inequality to see if it holds true.
- Interval Notation: Determine the domain of the equation or inequality and check if the solution lies within that domain.
- Graphing: Plot the equation or inequality on a graph and visualize the solution points.
Step 2: Examine Solutions Meticulously
After applying the methods above, you may have identified potential extraneous solutions. Now, it’s time for a thorough examination:
- Substitution: Substitute the solutions back into the original equation or inequality and verify if they hold true. If not, they are extraneous solutions.
- Interval Notation: Check if the solutions fall within the specified domain of the equation or inequality. Solutions outside the domain are extraneous.
- Graphing: Plot the solutions on the graph and see if they intersect with the graph of the equation or inequality. If not, they are extraneous solutions.
Step 3: Validate Final Solutions
Once you’ve checked for extraneous solutions, you have a refined set of solutions. To ensure accuracy, you can double-check their validity by:
- Plugging them back into the original equation or inequality to confirm they satisfy all conditions.
- Verifying that they lie within the specified domain (if applicable).
- Graphing the solutions and comparing them to the graph of the equation or inequality.
Remember, eliminating extraneous solutions is essential for obtaining accurate results. By following this step-by-step approach, you can confidently navigate the world of equations and inequalities, ensuring the integrity of your solutions.
Finding Extraneous Solutions: A Comprehensive Guide
When solving equations and inequalities, it’s crucial to be aware of the potential for extraneous solutions. These are solutions that satisfy the equation or inequality but don’t make sense within the given context or domain. Failing to identify and eliminate extraneous solutions can lead to incorrect results.
Methods for Finding Extraneous Solutions
There are several effective methods for detecting extraneous solutions:
Substitution
Simply substitute the suspected solution back into the original equation or inequality. If it doesn’t hold true, it’s an extraneous solution.
Interval Notation
Use interval notation to determine if solutions lie within the domain of the equation or inequality. If a solution falls outside the domain, it’s extraneous.
Graphing
Graph the equation or inequality and visualize the solutions. Extraneous solutions will often be points that lie outside the relevant graph.
Checking for Extraneous Solutions
Once you have potential solutions, follow these steps:
- Substitute each solution back into the equation or inequality.
- Check the domain using interval notation.
- Graph the equation or inequality if necessary.
Examples
-
Example 1: Solve the equation √(x – 3) = 2.
- Solution: x = 7. However, substituting 7 into the equation yields -1 = 2, which is false. Thus, 7 is an extraneous solution.
-
Example 2: Solve the inequality x2 – 4 < 0.
- Solution: -2 < x < 2. Using interval notation, we see that the domain is (-∞, -2) ∪ (-2, 2) ∪ (2, ∞). The solution set includes only (-2, 2), so there are no extraneous solutions.
Finding and eliminating extraneous solutions is essential for accurate results in solving equations and inequalities. By following the methods discussed, you can ensure that your solutions are both valid and meaningful within the given context.