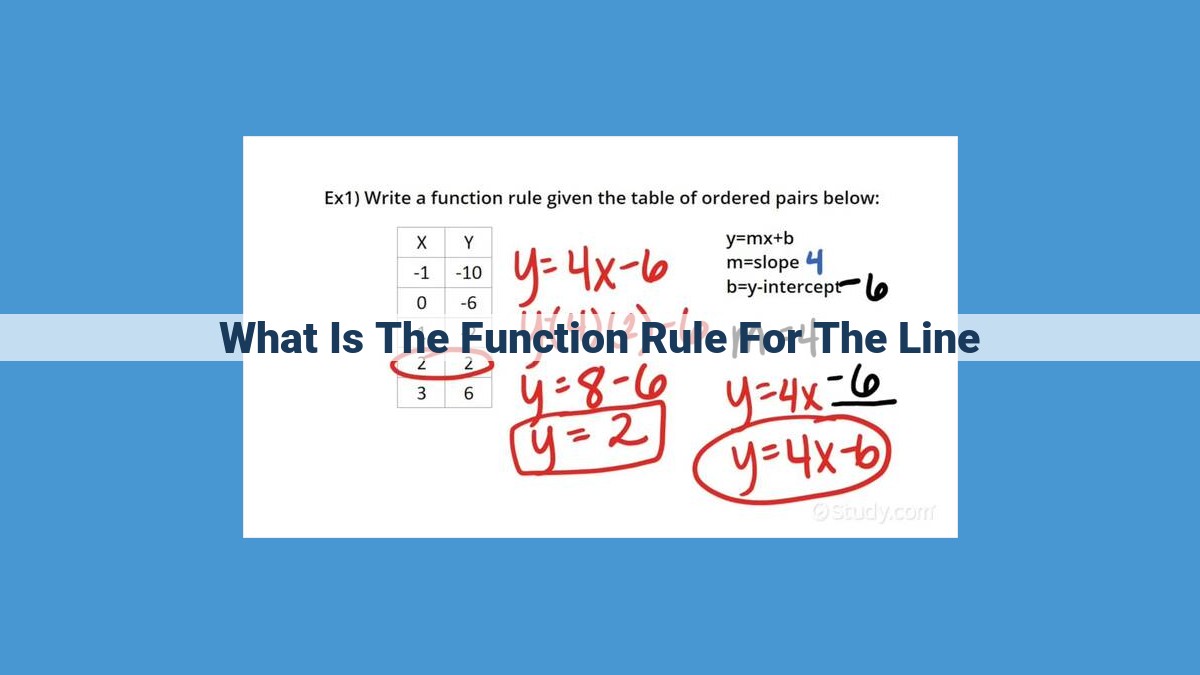
A function rule for a line expresses the relationship between x- and y-coordinates on the line in an equation. It is typically written in slope-intercept form: y = mx + b. Here, m represents the slope, or steepness of the line, and b represents the y-intercept, or the point where the line crosses the y-axis. By knowing both the slope and y-intercept, you can identify and draw any line on a graph. Understanding function rules for lines is essential for analyzing and solving problems involving linear relationships in various fields.
Unveiling the Secrets of Lines: A Journey Through Geometry
In the realm of geometry, lines hold a fundamental place, forming the very fabric of shapes and structures. Step into our tale and unravel the enigmatic world of lines, starting with the very beginning – understanding what a line truly is.
Line: The Unending Path
A line, in its essence, is a one-dimensional shape, a concept that may seem abstract at first. Imagine an infinitely long, infinitely thin thread stretched out before your eyes. That is the embodiment of a line – a path that extends endlessly in both directions, devoid of any thickness or width. It’s a boundary, a guide, a path that leads us through the geometric landscape.
From Definition to Equation
Lines, though abstract, can be described through mathematical equations. An equation of a line captures the relationship between the coordinates of points that lie on that line. It’s like a fingerprint, unique to each line, defining its position and orientation in the coordinate plane.
Equation of a Line: Unveiling the Mathematical Essence of Lines
When we behold a line, we often perceive it as a simple shape, extending infinitely in both directions. However, from a mathematical standpoint, the equation of a line holds the key to unlocking the underlying relationship between points on that line.
The equation of a line is a mathematical statement that describes the set of all points that lie on the line. It provides a concise way to represent the line’s position and orientation within a coordinate system. The equation typically takes the form of y = mx + b, where y represents the vertical coordinate, x represents the horizontal coordinate, m is the slope of the line, and b is the y-intercept.
The slope of a line measures its steepness or slant. It is calculated as the change in y divided by the change in x between any two points on the line. A positive slope indicates an upward-sloping line, while a negative slope indicates a downward-sloping line. The y-intercept is the point where the line intersects the y-axis. It represents the value of y when x is equal to zero.
The equation of a line is a fundamental tool in geometry and algebra. It allows us to analyze lines, determine their properties, and solve geometric problems involving lines. By understanding the equation of a line, we gain a deeper appreciation for the mathematical beauty and functionality of lines in the world around us.
Slope: The Measure of a Line’s Inclination
Just like a mountain climber assesses the steepness of a trail, mathematicians use slope to gauge the incline of lines on a graph. Slope is a numerical value that quantifies how much the line rises or falls for every unit it moves to the right.
Calculating the slope is as simple as connecting the dots. By measuring the change in y (the vertical difference between two points on the line) and the change in x (the horizontal difference), we can pinpoint the slope. Using the formula slope = change in y/change in x, we determine the line’s steepness.
Take the example of a line that ascends 3 units vertically and moves 4 units to the right. The slope here is 3/4, indicating that the line rises 3 units for every 4 units it travels horizontally. In contrast, a line with a slope of -2/5 descends 2 units for every 5 units it moves to the right.
Understanding the Language of Slope:
- A positive slope means the line is slanting upwards from left to right.
- A negative slope indicates a downward slant from left to right.
- A slope of zero signifies a horizontal line, while a slope undefined represents a vertical line.
Comprehending slope is crucial for deciphering graphs and equations in many fields, including physics, economics, and engineering. It empowers us to analyze trends, make predictions, and solve problems relating to lines and their behavior.
Navigating the Y-Intercept: A Gateway to Line Equations
The world of lines is an intricate tapestry woven with mathematical threads. Among these threads, the y-intercept stands as a beacon, guiding us to a line’s intersection with the y-axis, the vertical axis that maps up and down values.
The y-intercept is defined as the value of y when x equals 0. In other words, it tells us where the line crosses the y-axis. Think of it as a landmark on the line, a point where the line touches down on the vertical axis.
Understanding the y-intercept is crucial for several reasons. First, it helps us visualize the line. If we know the y-intercept, we can immediately pinpoint where the line begins its journey along the y-axis. This is particularly useful when graphing lines, as it provides a starting point for sketching the line’s path.
Furthermore, the y-intercept plays a vital role in determining the line’s slope, another important measure of a line’s character. The slope tells us how steep the line is, and it’s directly related to the change in y divided by the change in x. By observing the y-intercept, we can get a sense of the line’s overall slope.
In the equation of a line, the y-intercept is represented by the constant term, the number that does not have a variable attached. For instance, in the equation y = 2x + 5, the y-intercept is 5. This means that when x is 0, the corresponding y-value is 5, which is the point where the line crosses the y-axis.
By comprehending the y-intercept, we gain insights into the behavior of lines, which are essential building blocks of geometry and algebra. It’s like holding a key that unlocks a deeper understanding of the mathematical realm, allowing us to navigate the intricate world of line equations with ease.
The Point-Slope Formula: A Journey to Understanding Line Equations
In the realm of mathematics, lines have a special significance, forming the foundation for constructing shapes and understanding relationships. One crucial aspect of working with lines is the ability to write their equations, and the point-slope form provides a simple and effective approach.
The point-slope formula, denoted as y – y1 = m(x – x1), allows us to write the equation of a line when given a point (x1, y1) that lies on the line and its slope (m). By understanding how to apply this formula, we can unlock the secrets of line equations and their applications.
Imagine yourself as a traveler on a mathematical expedition, embarking on a quest to explore the point-slope formula. Along the way, you encounter various landscapes and challenges, but with each step, you gain a deeper comprehension of this fundamental concept.
The Landscape of Coordinates
Every line exists within a coordinate plane, a two-dimensional space where each point is defined by an x-coordinate and a y-coordinate. The point (x1, y1) represents a specific location on the plane, akin to a signpost indicating your starting point.
The Measure of Steepness
Slope, represented by the letter m, is the measure of a line’s steepness or inclination. It describes how much the line rises or falls as you move from left to right. A positive slope indicates an upward-sloping line, while a negative slope signifies a downward-sloping line.
The Equation’s Anatomy
The point-slope formula comprises two sides separated by an equal sign. On the left side, you have y – y1, which represents the vertical change or difference between the y-coordinate of any point on the line and the y-coordinate of the given point (x1, y1).
On the right side, you have m(x – x1), which captures the horizontal change or difference between the x-coordinate of any point on the line and the x-coordinate of the given point (x1, y1). Multiplying the slope (m) by this horizontal change indicates how much the line rises or falls for every unit move in the x-direction.
Assembling the Equation
To write the equation of a line using the point-slope formula, simply substitute the values of (x1, y1) and m into the formula. For instance, if we have a line that passes through the point (2, 3) and has a slope of 2, the equation of the line would be:
y – 3 = 2(x – 2)
Empowering Applications
The point-slope formula finds applications in various mathematical disciplines and real-world scenarios. For example, it can be used to:
- Find the equation of a tangent line to a curve at a given point
- Model the relationship between two variables in a linear system
- Predict the trajectory of a moving object
The point-slope formula is a gateway to understanding line equations and their role in mathematics. By mastering this formula, you can navigate the landscape of coordinate geometry with confidence and apply your knowledge to solve problems in various contexts. Remember, the point-slope formula is a powerful tool that empowers you to unravel the secrets of lines, one equation at a time.
Slope-Intercept Form
- Introduce the slope-intercept form (y = mx + b).
- Explain how the slope and y-intercept are easily identified in this form.
Unveiling the Secrets of Line Equations: Slope-Intercept Form
In the realm of mathematics, lines play a crucial role in describing relationships and patterns. To fully grasp their essence, we need to delve into the concept of line equations.
One of the most common and useful forms of line equations is the slope-intercept form, expressed as:
y = mx + b
where:
- y is the dependent variable (the value that varies based on the value of x)
- m is the slope, which measures the steepness of the line
- x is the independent variable (often representing time or distance)
- b is the y-intercept, which represents the point where the line crosses the y-axis
The slope is an essential characteristic of a line. It indicates how quickly the y-coordinate changes as the x-coordinate increases. A positive slope means the line is rising from left to right, while a negative slope indicates a line falling from left to right.
The y-intercept is equally important. It tells us the value of y when x is 0. This point gives us a quick reference for where the line intersects the y-axis.
By knowing both the slope and the y-intercept, we can easily sketch a line. The slope tells us how steep the line is, and the y-intercept gives us a starting point.
The slope-intercept form is particularly useful for analyzing linear functions. The equation of a linear function is always in slope-intercept form, making it easy to identify the slope and y-intercept. This information can then be used to make predictions and draw conclusions about the function’s behavior.
In essence, the slope-intercept form provides us with a powerful tool for understanding and working with lines in mathematics and beyond. Whether you’re analyzing data, solving equations, or modeling real-world phenomena, the slope-intercept form will always be a reliable guide.
Understanding the Function Rule for a Line: A Path to Clarity
In the realm of mathematics, lines play a pivotal role in describing the relationship between two variables. They serve as a cornerstone for understanding various concepts, from geometry to algebra. And one crucial aspect of understanding lines lies in comprehending their function rules.
A function rule, in its essence, is an equation that captures the connection between the x- and y-coordinates on a line. It provides a precise description of the line’s behavior and allows us to make predictions about the y-value for any given x-value (or vice versa).
The most common form of a function rule for a line is the slope-intercept form, written as:
y = mx + b
where:
- y represents the y-coordinate
- x represents the x-coordinate
- m represents the slope of the line
- b represents the y-intercept
The slope (m) measures the steepness or slant of the line. It indicates how much the line rises (or falls) for every unit increase in x. The y-intercept (b), on the other hand, represents the point where the line crosses the y-axis. It tells us the value of y when x is equal to zero.
To understand the function rule for a line, let’s delve into a simplified example. Consider the line with the equation y = 2x + 1. This equation tells us that for every increase of 1 unit in x, the y-coordinate increases by 2 units. The slope of this line is 2, indicating that it rises steeply. The y-intercept is 1, meaning that the line crosses the y-axis at the point (0, 1).
Function rules for lines are not merely abstract concepts; they have practical applications across various fields. In physics, lines can represent the relationship between speed and time or distance and velocity. In economics, lines can depict the relationship between supply and demand or price and quantity. By understanding the function rule for a line, we gain the ability to analyze and interpret real-world phenomena more effectively.
In conclusion, the function rule for a line is a fundamental aspect of understanding lines and their applications. The slope-intercept form provides a straightforward way to describe the relationship between the x- and y-coordinates on a line. By grasping this concept, we empower ourselves to navigate the world of mathematics and beyond with confidence.