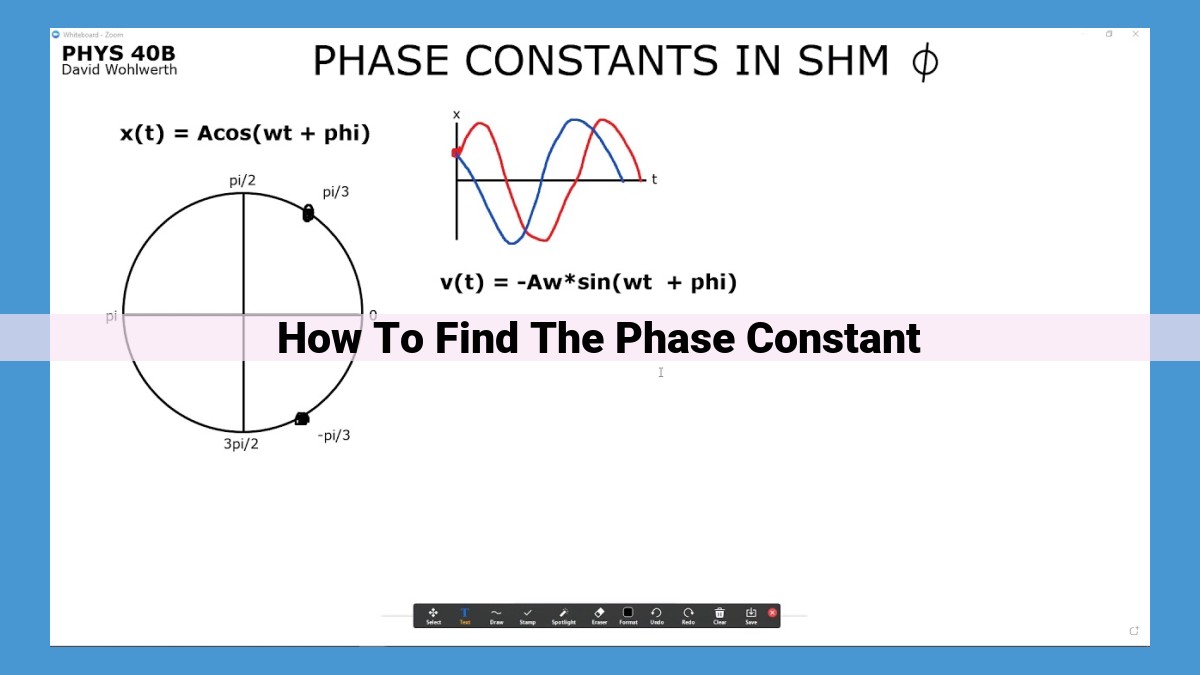
To find the phase constant, first understand oscillatory motion and the role of time in sinusoidal motion. The phase constant determines the starting point of oscillation and is related to the initial phase and phase shift. Use angular displacement to calculate the initial phase and phase shift, which are also related to the frequency and period. Remember that frequency and period are inversely proportional, meaning high frequency corresponds to a short period. By considering these concepts, you can effectively determine the phase constant.
Understanding Oscillatory Motion
- Define the key concepts of angular frequency, frequency, and period.
- Explain how these parameters describe the repetitive nature of oscillations.
Understanding Oscillatory Motion: Unraveling the Rhythmic Dance of Nature
Oscillations, the rhythmic back-and-forth motions we encounter throughout nature, play a crucial role in our understanding of the physical world. From the beating of our hearts to the vibrations of a musical string, oscillations govern countless phenomena. To delve into this intriguing realm, let’s explore the fundamental concepts of angular frequency, frequency, and period.
Angular frequency (ω) measures the rate of oscillation per unit of time. It is expressed in radians per second (rad/s) and represents the speed at which an object completes one full cycle of oscillation.
Frequency (f), on the other hand, refers to the number of oscillations completed in one second, measured in hertz (Hz). A higher frequency indicates that the oscillation is occurring faster.
Period (T), in contrast, is the duration of one complete oscillation, measured in seconds (s). It is the reciprocal of frequency, meaning that a shorter period corresponds to a higher frequency and vice versa.
These three parameters, angular frequency, frequency, and period, work together to describe the repetitive nature of oscillations. They provide a precise and quantifiable way to analyze and understand the rhythmic motions that surround us.
The Role of Time in Sinusoidal Motion
In the realm of oscillatory motion, time plays a pivotal role, orchestrating the rhythmic dance of waves and vibrations. Oscillations, whether it’s the swinging of a pendulum or the throbbing of a guitar string, are inherently repetitive, and time serves as the maestro that dictates their cadence.
Sinusoidal equations, with their undulating curves, provide a mathematical representation of these oscillations. Time takes center stage as the independent variable, acting as the conductor that determines the position of the object at any given moment.
One of the most fundamental concepts in oscillatory motion is period, the time it takes for the object to complete one full oscillation. Imagine a child on a swing: the time it takes for them to go from the highest point to the lowest point and back again is their period.
Phase shift, on the other hand, refers to the horizontal displacement of the wave from its origin. It’s like the starting point of the oscillation, much like the angle at which the child starts their swing. By shifting the phase, we can adjust the timing of the oscillation, effectively delaying or advancing its motion.
Phase Constant: The Starting Point of Oscillation
- Define phase constant as a constant that determines the starting point of oscillation.
- Relate phase constant to initial phase and phase shift.
Phase Constant: The Starting Point of Oscillation
In the realm of oscillatory motion, understanding the role of the phase constant is crucial. This constant acts as the gatekeeper, determining the starting point of oscillation, the point from which the dance of periodic motion commences.
To grasp the concept of phase constant, let’s delve into two related terms: initial phase and phase shift. The initial phase signifies the position of the oscillating object at the onset of motion, while the phase shift represents the horizontal displacement of the wave from its origin. The phase constant, in essence, is the bridge between these two concepts.
Imagine a pendulum swinging rhythmically, marking the passage of time. The phase constant is like the initial push that sets the pendulum in motion. It determines the pendulum’s starting angle, dictating its journey from its highest point to its lowest and back again.
The initial phase, often denoted by the symbol “φ₀,” is the angle at which the object begins its oscillation. The phase shift, represented by “δ,” is the angle by which the wave has been displaced from its original position.
The phase constant, symbolized by “θc,” establishes a relationship between the initial phase and phase shift:
θc = φ₀ - δ
By adjusting the phase constant, we can effectively shift the starting point of oscillation. This has significant implications in various fields, such as electronics, where precise control over the timing of signals is paramount.
Angular Displacement and Phase Shift
In the realm of oscillatory motion, angular displacement plays a crucial role in understanding the object’s position at any given moment. It refers to the angle formed between a reference line and the object’s current position. This angle is measured in radians.
The initial phase, also known as the phase constant, determines the object’s starting point in its oscillatory journey. It establishes the angle at which the object begins its oscillation relative to the reference line.
The phase shift represents the horizontal displacement or shift of the wave from its original position. It indicates the extent to which the wave has moved along the time axis. Both initial phase and phase shift can be calculated using angular displacement.
Imagine a pendulum swinging back and forth. As the pendulum moves from its equilibrium position, it forms an angle with the vertical reference line. This angle is the angular displacement. The initial phase is the angle at which the pendulum starts its swing, while the phase shift is the angle by which the pendulum has shifted from its initial position.
By analyzing angular displacement, we gain insights into the object’s position and movement within the oscillatory system. Understanding these concepts is essential for unraveling the mysteries of waves, pendulums, and other oscillatory phenomena.
Frequency and Period: An Inverse Dance
In the realm of oscillations, two fundamental quantities reign supreme: frequency and period. These parameters describe the rhythmic nature of a system’s motion, providing key insights into its behavior. In this section, we’ll explore the intriguing inverse relationship between frequency and period.
Imagine a child swinging on a playground. The faster they swing, the more times they complete an oscillation in a given amount of time. This corresponds to a higher frequency. On the other hand, if they swing more slowly, the time it takes to complete one swing, or the period, becomes longer.
Mathematically, this inverse relationship can be expressed as:
Frequency = 1 / Period
In other words, as frequency increases, period decreases. Conversely, when period increases, frequency decreases. It’s a delicate balance, where one cannot exist independently of the other.
To illustrate this, consider two pendulums, one with a short period (and high frequency) and the other with a long period (and low frequency). As time progresses, you’ll notice the pendulum with the shorter period swinging more rapidly, completing more oscillations in the same time frame compared to its longer-period counterpart.
Understanding the inverse relationship between frequency and period is crucial in various applications. For instance, in music, the frequency of a note determines its pitch, while in physics, the resonant frequency of an object can influence its response to external vibrations. By harnessing this knowledge, we gain valuable insights into the behavior of oscillating systems, both natural and man-made.