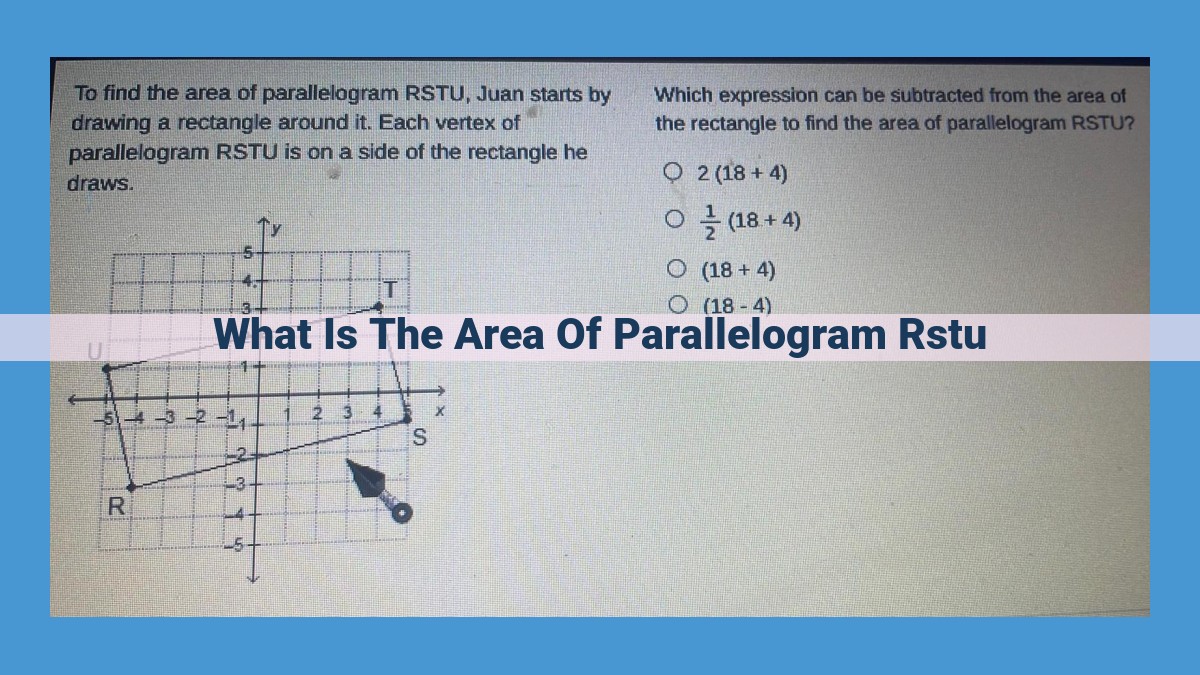
To determine the area of parallelogram RSTU, we need to identify its base and height, which represent the length and width, respectively. Using the formula Area = base × height, we can calculate the area. The diagonals of a parallelogram divide it into two congruent triangles, with their intersection point forming the midpoint of each diagonal. Understanding these properties and the area calculation method is essential for solving related problems and applying parallelogram concepts in practical applications.
- Discuss the definition, characteristics, and related concepts of parallelograms.
- Explain the concept of area, its units of measurement, and the formula for calculating it.
Understanding Parallelograms and Area
In the realm of geometry, parallelograms stand as unique quadrilaterals with distinct characteristics. They are characterized by their four sides, which are arranged in pairs of parallel lines, as their name suggests. Often represented by the humble rectangle or the more eccentric rhombus, parallelograms embody the essence of duality, mirroring each other across a diagonal axis.
Delving into the Concept of Area
At the heart of geometry lies the concept of area, a quantifier of the extent occupied by a two-dimensional figure. Measured in units like square meters or square feet, area plays a crucial role in understanding the size and proportions of geometric shapes. For parallelograms, the formula for calculating area is straightforward: (base) × (height).
Unveiling the Base and Height of Parallelogram RSTU
The base of a parallelogram is like the foundation upon which it rests. The height, on the other hand, represents the vertical distance from the base to the opposite side. In the case of parallelogram RSTU, we embark on a geometric adventure to identify these key dimensions, unraveling the secrets of its area.
Applying the Formula to Calculate RSTU’s Area
Equipped with the knowledge of base and height, we embark on the next leg of our quest: applying the area formula. Like a skilled alchemist, we plug the values of base and height into the magical formula, confident in the transformative power of mathematics. With each step of the calculation, we draw closer to unlocking the hidden treasure of RSTU’s area.
The Role of Diagonals and Beyond
Diagonals, the enigmatic lines that bisect parallelograms, play a pivotal role in our understanding. They not only divide the parallelogram into two congruent triangles but also bear a harmonious relationship with the base and height. Their insights extend beyond the realm of area calculation, offering valuable applications in the wider context of real-world scenarios.
Identifying the Base and Height of Parallelogram RSTU
In our journey to unravel the secrets of parallelograms, we now embark on the crucial task of identifying their base and height. These two measurements hold the key to unlocking the area of our parallelogram, RSTU.
Step 1: The Base – A Sturdy Foundation
Imagine the parallelogram RSTU as a stable platform resting on the ground. The base is the bottom side of this platform, the sturdy foundation upon which the parallelogram stands. In our diagram, line RS represents the base.
Step 2: The Height – A Vertical Reach
Now, visualize a perpendicular line rising from the base towards the opposite side of the parallelogram. The height measures the vertical distance between the base and the opposite side. In our diagram, line RT represents the height of parallelogram RSTU.
Examples to Enhance Clarity
To illustrate these concepts, let’s consider some examples:
- In a rectangular garden, the base would be the length of the garden, and the height would be the width.
- In a parallelogram-shaped window frame, the base could be the bottom side, while the height could be the distance from the bottom to the top.
Remember, the base and height are perpendicular to each other, forming right angles. By accurately identifying these two measurements, we lay the groundwork for calculating the area of parallelogram RSTU.
Applying the Area Formula to Calculate the Area of Parallelogram RSTU
Now, we’re ready to calculate the area of parallelogram RSTU using the formula we learned: Area = Base x Height.
First, let’s identify the base and height of the parallelogram. The base is the length of the side that is parallel to the height. In parallelogram RSTU, the base is RS. The height is the perpendicular distance from the base to the opposite side. In parallelogram RSTU, the height is TU.
Once we have identified the base and height, we can plug the values into the formula:
Area = RS x TU
Let’s assume that the length of the base RS is 6 units and the height TU is 4 units. Substituting these values into the formula, we get:
Area = 6 units x 4 units
Area = 24 square units
Therefore, the area of parallelogram RSTU is 24 square units.
Remember, when calculating the area of a parallelogram, it’s crucial to ensure that the units of measurement for both the base and height are the same. In this case, both the base and height are in units, so the area is expressed in square units.
Role of Diagonals and Additional Considerations in Parallelogram Area Calculations
As we delve deeper into the world of parallelograms, let’s unravel the role of their diagonals and explore some fascinating insights and practical applications.
Diagonals: Dividing Parallelograms into Triangular Halves
Diagonals are line segments that connect opposite vertices of a parallelogram. Interestingly, they divide the parallelogram into two congruent triangles. This property is particularly useful in understanding the relationship between the parallelogram’s area and its base, height, and diagonals.
Relationship between Diagonals, Base, and Height
The diagonals of a parallelogram intersect at a single point, and their lengths are related to the base and height of the parallelogram. Knowing this relationship allows us to calculate the area of the parallelogram without explicitly having the height or base.
Applications in Real-World Contexts
The concept of parallelogram area finds numerous applications in real-world scenarios:
- Architecture: Calculating the area of walls, ceilings, or roofs that can be modeled as parallelograms.
- Land Surveying: Determining the area of land plots or fields that are often shaped as parallelograms.
- Fabric Design: Designing symmetrical patterns or fabrics that utilize the area properties of parallelograms.
- Engineering: Calculating the surface area of items with parallelogram-shaped components, such as solar panels or bridges.
By understanding the role of diagonals and additional considerations, we gain a deeper appreciation for the properties of parallelograms and their practical significance in various fields.