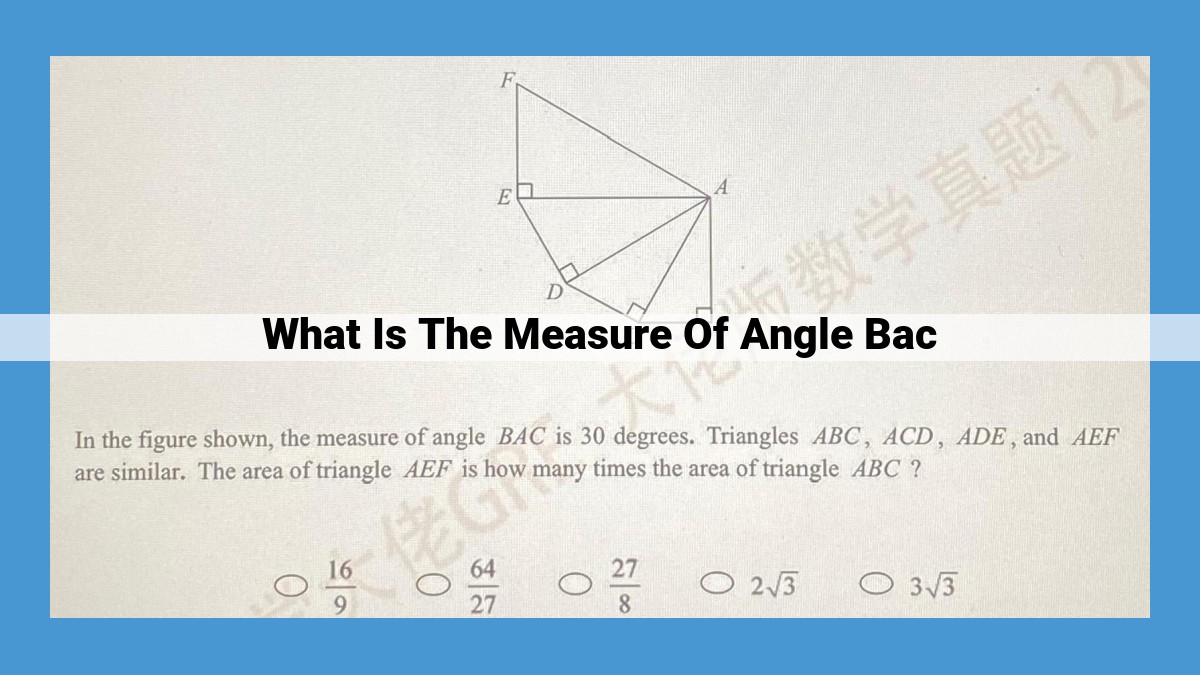
Angle BAC is measured using a protractor. Position the protractor’s center on point B, aligning its baseline with line segment AB. Mark the point where line segment AC intersects the protractor’s scale. The angle measure, in degrees, is indicated by the marked point on the scale.
Define an angle as formed by two rays sharing a common endpoint.
Angle: The Shaping Force of Our World
Have you ever wondered about the hidden angles that govern the world around us? From the gentle curve of a leaf to the soaring height of a building, angles shape our lives in countless ways. Join us on a journey to unravel the enigmatic world of angles, where lines, rays, and points intertwine to create the intricate fabric of our existence.
Chapter 1: Defining the Angle
An angle is a fundamental geometric concept that describes the amount of turn or rotation between two intersecting rays. Imagine two rays emanating from a common point, like two spokes of a wheel. The space between these rays is what we call an angle.
Chapter 2: Measuring Angles with a Protractor
To quantify the size of an angle, we use a handy tool called a protractor. This semicircular device is marked with precise degree measures. Simply align the flat edge of the protractor with one ray and note the degree reading where the other ray intersects the scale.
Chapter 3: The Components of an Angle
To delve deeper into the anatomy of an angle, we need to understand its component parts. A line is a straight path that extends indefinitely in both directions. A line segment is a part of a line bounded by two points. And a point is a fixed location in space, the meeting point of two rays.
Chapter 4: Measuring Angle BAC
Let’s put our knowledge to practice by measuring angle BAC, formed by the intersection of two line segments, AB and AC. Align the center of the protractor with point A, then place the flat edge along AB. Now, read the degree measure where AC intersects the scale. This value represents the angle BAC.
Understanding angles is essential for deciphering the complexities of our world. From architecture and engineering to astronomy and navigation, angles play a pivotal role in shaping our lives. By mastering the measurement of angles, we gain a deeper appreciation for the hidden symmetries and patterns that surround us. So, let us embrace the world of angles, where geometry unveils the beauty and precision of our existence.
Delving into the Secrets of Angles: A Guide to Angle Measurement
From the towering heights of skyscrapers to the intricate patterns of nature, angles shape our world and are integral to our understanding of geometry. Embark on a journey to unravel the mysteries of angles, exploring their definition, measurement, and the essential components that define them.
Unveiling the Nature of Angles
An angle is the measure of the space between two rays that share a common endpoint, like the hands of a clock pointing towards numbers. We encounter angles everywhere we turn, from the slope of a hill to the angle of a jumping bean.
The Protractor: A Tool for Angle Measurement
Introducing the protractor, the trusty device that empowers us to measure angles with precision. This half-circle shaped tool features a scale marked in degrees and can be aligned along the rays of an angle to determine its measure. By noting the intersection between the scale and the ray, we uncover the angle’s magnitude.
Components of an Angle: The Building Blocks
Now, let’s zoom in on the building blocks of angles. Points serve as the fixed locations where rays meet. Line segments are straight paths that connect two points. And lines are infinitely extending paths without endpoints. These components orchestrate to form the angles we encounter.
Measuring Angle BAC: A Step-by-Step Guide
To measure angle BAC, formed by intersecting line segments AB and AC, follow these steps:
- Align the protractor’s center with point B, the angle’s vertex.
- Rotate the protractor until one of its scales coincides with line segment AB.
- Note the point where the other scale intersects line segment AC.
- The degree measurement indicated at this intersection is the measure of angle BAC.
Understanding angles and their measurement is fundamental for unlocking the secrets of geometry and beyond. From architecture to engineering, from physics to everyday problem-solving, the application of angle measurement is vast and indispensable. Embrace the knowledge of angles and let it guide you towards a deeper understanding of the world around us.
Measuring Angles with a Protractor: A Beginner’s Guide
Understanding angles is essential not only in mathematics but also in various fields such as engineering, architecture, and carpentry. Accurately measuring angles is crucial for precision and accuracy in these disciplines. One of the most common and accessible tools for angle measurement is a protractor.
In this blog post, we will embark on a step-by-step journey into the world of angle measurement with a protractor. We will begin with the basics and gradually progress to more advanced techniques, ensuring that every reader can grasp this fundamental concept.
Aligning the Protractor
Before we dive into measuring angles, let’s familiarize ourselves with the protractor. This handy tool consists of a circular or semicircular scale marked with degree markings. The center point of the protractor represents the vertex of the angle we wish to measure.
To align the protractor, place the center point of the protractor precisely on the vertex of the angle. Ensure that the baseline of the protractor, usually the straight edge, aligns perfectly with one of the rays forming the angle.
Noting the Measurement on the Scale
Once the protractor is correctly aligned, it’s time to note the measurement on the scale. Locate the point where the other ray of the angle intersects the protractor. Read the degree measurement corresponding to this point on the scale.
If the angle is less than 180 degrees, read the measurement on the inner scale of the protractor. For angles greater than 180 degrees, use the outer scale.
Remember, accuracy is key when reading the scale. Pay close attention to the smallest divisions on the protractor, which are typically one degree or half a degree.
By following these simple steps, you can confidently measure angles with a protractor. This skill will empower you to tackle countless practical applications in the future.
Understanding Angle Measurement: A Simple Guide
In the world of geometry, angles play a crucial role in describing shapes, paths, and spatial relationships. An angle is formed by two rays that share a common endpoint. Imagine a clock’s hands pointing at different numbers: the space between the hands forms an angle.
To accurately measure angles, we rely on a tool called a protractor. This handy device features a half-circle or circle with markings that indicate the angle’s measurement. Placing the protractor’s baseline along one ray and aligning its center with the endpoint, we can easily read the measurement on the scale.
Before we delve into measuring angles, let’s understand the basic building blocks:
Line Segments: Connecting Points
Line segments are straight paths that connect two points. Think of a path between two cities on a map, or a piece of string you stretch taut. Line segments have definite endpoints and a specific length.
Lines: Extending Infinitely
Lines are straight paths that extend infinitely in both directions. They have no endpoints and represent an endless path. In geometry, lines are often used to create shapes and describe relationships between points.
Points: Fixed Locations
Points are fixed locations in space. They have no size or shape, and are often represented by a small dot. Points are the building blocks of geometry and serve as endpoints for line segments and rays.
Understanding these components will help us navigate the world of angles with ease. Stay tuned for our next section, where we’ll explore how to measure specific angles using a protractor.
Define lines as straight paths extending infinitely.
What’s an Angle, and How Can I Measure It?
Picture this: You’re standing at a crossroads, with two roads leading off in different directions. The space between these roads forms what’s known as an angle, a geometric shape formed by two rays (those straight lines radiating out like spokes from the intersection) that share a common starting point, called the vertex.
Now, let’s say you want to measure how wide this angle is. Enter the protractor, a handy tool that looks like a half-circle with markings along the curved edge. Align the straight edge of the protractor with one of the rays, with the vertex at the center mark. Then, read the number on the scale where the other ray intersects the protractor. That’s your angle measurement in degrees.
The Building Blocks of Angles
Every angle has three essential components:
- Line segments are paths that connect two points.
- Lines are straight paths that extend infinitely in one direction. They’re like roads leading off into the horizon.
- Points are fixed locations in space, like the intersection where your roads meet.
Measuring Angle BAC
Let’s say we want to measure the angle BAC, formed by the intersection of line segments AB and AC. Here’s a step-by-step guide:
- Place the protractor’s vertex at the intersection of AB and AC.
- Align the protractor’s straight edge with line segment AB.
- Note the measurement where AC intersects the protractor.
- That’s your angle BAC measurement in degrees.
Why Angles Matter
Understanding angles is crucial for tasks ranging from navigation to engineering. From measuring the angle of a baseball pitcher’s throw to designing the shape of a bridge, angles play a vital role in our everyday world.
So, remember, next time you’re at a crossroads or measuring a construction project, angles are the key to understanding the shape and direction of things around you.
Understanding Angles: From Theory to Measurement
Angle Basics: A Ray of Two
What’s an angle? Picture two rays shooting out from the same point like beams of light. The point they share is known as the vertex, and the space between the rays is the angle.
Protractor: Your Angle-Measuring Buddy
Measuring angles is a breeze with a protractor. This handy device resembles a semicircle with markings that indicate the degrees of an angle. Align the protractor’s base line with one ray and note the reading on the scale where the other ray intersects.
Unraveling the Components: Lines, Segments, Points
An angle is like a stage where three actors play their roles:
- Line segments: Imagine paths connecting points, like line segments AB and AC that form an angle.
- Lines: Picture a straight path extending infinitely, such as line m that passes through the vertex.
- Points: These are like fixed locations on our stage, like point B where the rays intersect.
Measuring Angle BAC: A Step-by-Step Guide
Let’s measure angle BAC formed by intersecting line segments AB and AC.
- Align the Protractor: Place the protractor’s base line along AB.
- Note the Reading: Where ray AC intersects the protractor’s scale, read the angle measurement. That’s the value of angle BAC.
The Significance of Angles: A World at Sharp Perspectives
Understanding angles is no mere academic exercise. From architecture to engineering, navigation to graphic design, the ability to measure angles finds practical application in countless fields. So, embrace the world of angles and become a pro at measuring them!
Angle Measurement: A Comprehensive Guide
Embark on an exciting journey into the world of angles, where we’ll uncover their secrets and master the art of measuring them with precision.
What’s an Angle?
Imagine two roads crossing at an intersection. The point where they meet is called the vertex. The paths extending from the vertex are rays. The space between these rays forms the angle.
Measuring with a Protractor
Meet the protractor, our trusty tool for angle measurement. Align its center mark with the vertex and the zero mark with one ray. Note the reading where the other ray intersects the scale. Presto, you’ve got your angle measure!
Components of an Angle
Just as roads connect cities, line segments connect points. When line segments extend indefinitely, they become lines. And points themselves are like tiny dots in space.
Measuring Angle BAC
Let’s focus on angle BAC, formed by intersecting line segments AB and AC. Using our protractor, we place it at B, align the base with AB, and read the angle at C. Voilà, we’ve conquered angle BAC!
Understanding angles is crucial for navigating our world. From architects designing buildings to engineers building bridges, angle measurement underpins countless practical applications. Embrace this knowledge and unlock the wonders of geometry!
Understanding Angles: A Comprehensive Guide with Step-by-Step Protractor Instructions
What is an Angle?
Angles are geometric figures formed when two rays, or lines with a common endpoint, come together. Imagine yourself standing at a crossroad where two streets intersect. The angle is the area where the streets join.
Measuring Angles with a Protractor
Introducing the Protractor:
The protractor is your trusty companion in measuring angles. It’s a semicircular device with a degree scale printed along the arc.
Aligning the Protractor:
When measuring an angle, carefully align the flat edge of the protractor along one ray, ensuring the endpoint lies at the center point.
Reading the Measurement:
Now, trace the other ray along the protractor’s curved edge. At the point where the ray intersects the scale, you’ll find the angle’s measurement in degrees.
The Components of an Angle
Line Segments and Lines:
Line segments are paths that connect two points. When these line segments extend endlessly in one direction, they become lines. Think of them as paths without beginning or end.
Points:
Points are specific locations in space. Imagine a tiny dot on a piece of paper. That’s a point.
Measuring Angle BAC
Identifying the Angle:
Angle BAC is formed by the intersection of line segments AB and AC. It’s named after the three points that define it.
Step-by-Step Protractor Instructions:
- Align the Base: Place the flat edge of the protractor along line segment AB, with B at the center point.
- Trace the Ray: Trace line segment AC along the curved edge of the protractor.
- Note the Measurement: Find the point where AC intersects the degree scale. The value you see is the measure of angle BAC.
Understanding angles is crucial in various fields, from architecture to physics. By mastering angle measurement with a protractor, you’ll unlock a powerful tool for understanding the world around you. It’s like having a secret decoder ring for geometric mysteries!
Understanding Angles: A Guide to Measuring and Their Importance
What is an Angle?
An angle is formed by two rays sharing a common endpoint. This endpoint is called the vertex.
Measuring Angles with a Protractor
A protractor is a tool used to measure angles. To use a protractor, align it with the vertex of the angle and note the measurement on the scale.
The Components of an Angle
An angle has three basic components:
- Line Segments: Paths connecting points.
- Lines: Straight paths extending infinitely.
- Points: Fixed locations in space.
Measuring Angle BAC
To measure angle BAC, formed by intersecting line segments AB and AC, follow these steps:
- Align the protractor with the vertex B.
- Read the measurement on the scale at the point where AB and AC intersect.
Importance of Understanding Angles and Their Measurement
Understanding angles and their measurement is crucial in various fields. In architecture, it is used to design buildings and calculate the angles of roofs. In engineering, it is used to construct bridges and machines. In geometry, it is used to classify shapes and calculate their areas.
Even in our daily lives, we encounter angles. From the angle of a staircase to the angle of a camera lens, understanding angles helps us make informed decisions. By mastering this concept, you’ll unlock a world of possibilities where angles play a vital role.
Understanding Angles: A Guide to Measuring and Their Practical Applications
In geometry, angles are essential concepts that describe the spatial relationship between two intersecting lines or rays. Whether you’re an aspiring architect, a budding engineer, or simply curious about the world around you, understanding angles and their measurement is crucial.
What are Angles?
An angle is formed when two rays share a common endpoint. Imagine opening a book and the angle created by the two pages is an example of an angle.
Measuring Angles with a Protractor
To measure angles precisely, we use a device called a protractor. It’s a semicircular tool with a scale that indicates the degree measure of angles. By aligning the protractor’s base with one ray and its center with the endpoint, you can read the corresponding angle measurement on the scale.
Components of an Angle
Understanding the components of an angle is key to accurately measuring it. The vertex is the common endpoint where the rays meet, and the rays are the line segments extending from the vertex. Additionally, lines are straight paths that extend infinitely, while points represent fixed locations in space.
Measuring Angle BAC
Let’s say we have an angle formed by intersecting line segments AB and AC, denoted as angle BAC. To measure this angle using a protractor:
- Place the protractor’s base on line AB.
- Align the vertex of the protractor with point B.
- Note the measurement on the protractor’s scale where line AC intersects the scale.
- This measurement represents the degree measure of angle BAC.
Practical Applications of Measuring Angles
Measuring angles has numerous practical applications across various fields:
- Architecture: Architects use angles to determine the slopes of roofs, design symmetrical structures, and ensure stability.
- Engineering: Engineers rely on angles to calculate the strength of bridges, design aircraft wings, and optimize machinery for efficiency.
- Construction: Angles are crucial for framing houses, aligning pipes, and ensuring the accuracy of various construction projects.
- Navigation: Sailors and pilots use angles to determine their position, navigate routes, and estimate distances.
- Everyday Life: We unconsciously encounter angles in our daily lives, such as when determining the angle of a sunbeam, setting the tilt of a picture frame, or adjusting the angle of a speaker for optimal sound distribution.
Understanding angles and their measurement is essential for navigating our world and unlocking its countless possibilities. From grand architectural feats to precision engineering and our everyday interactions, angles play a vital role in our lives. Embracing the knowledge of angles empowers us to design, build, navigate, and engage with the world in new and meaningful ways.