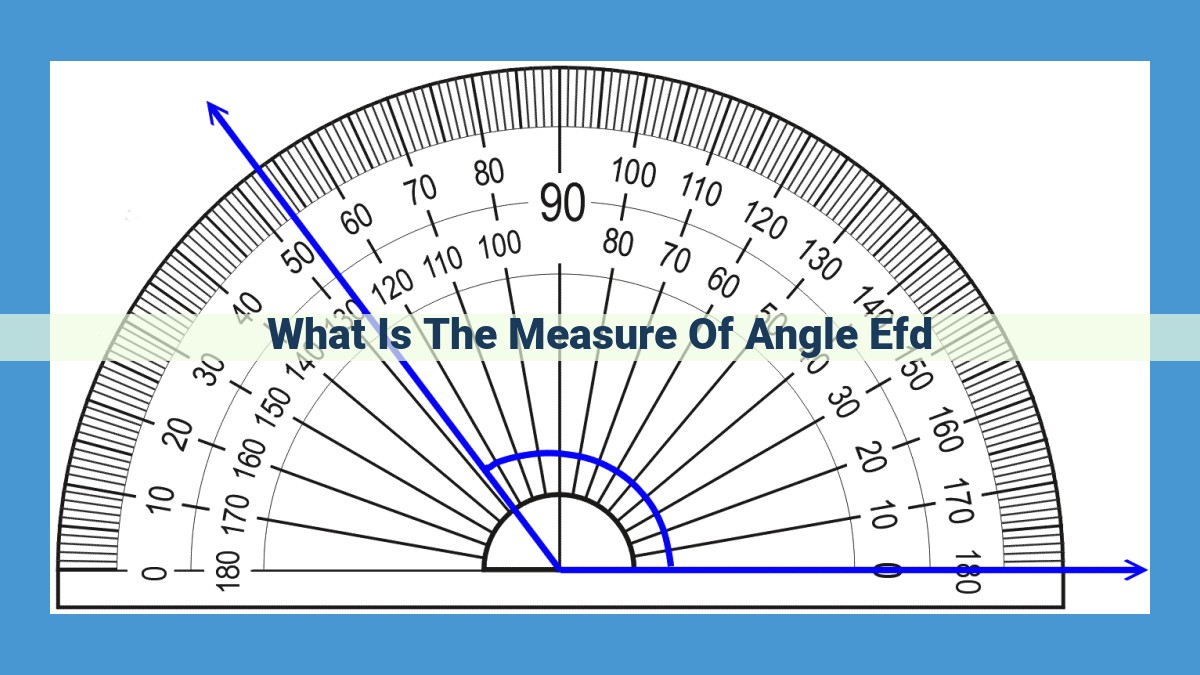
To measure the measure of angle EFD using a protractor, align the protractor’s center point with the vertex of angle EFD and its zero mark with one of the angle’s rays. Mark the point where the other ray intersects the protractor’s scale. The number of degrees or minutes between the zero mark and the marked point represents the measure of angle EFD. For accurate measurements, ensure the protractor is aligned correctly and the markings are precise.
Understanding Angle Measurement: The Key to Unlocking Spatial Relationships
In the realm of geometry, angles play a pivotal role in describing and quantifying the relationships between lines and surfaces. Angle measurement is the intricate art of determining the amount of rotation between two rays or lines that meet at a common endpoint, known as the vertex.
Defining Angle Measurement
An angle is best understood as the amount of spatial separation between two lines that meet at a single point. It is measured in degrees or radians, with the degree being the most commonly used unit. A full rotation, where one line turns completely around the vertex, is equivalent to 360 degrees or 2π radians.
The Importance of Measuring Angles
Angle measurement is a fundamental skill in various fields, including:
- Architecture: Designing buildings with precise angles ensures stability and aesthetic appeal.
- Engineering: Measuring angles in bridges, machines, and other structures is crucial for their structural integrity.
- Navigation: Pilots and sailors use angles to determine their location and course.
- Sports: Angles play a significant role in predicting ball trajectories in sports such as golf, tennis, and soccer.
Protractor: The Essential Tool for Measuring Angles
In the realm of geometry and everyday life, angles play a pivotal role. Angle measurement allows us to quantify the extent of a rotation or deviation between two lines. To embark on this precise endeavor, we turn to a trusty tool: the protractor.
A Glimpse of the Protractor
Imagine a semicircular device adorned with intricate markings. These markings, called degree measures, serve as a roadmap for deciphering angles. Each degree represents 1/360th of a complete circle, providing us with a precise scale for measuring the size of angles.
Unveiling the Art of Angle Measurement
To harness the power of a protractor, follow these expert steps:
-
Position the protractor: Align the flat base of the protractor with one of the arms of the angle. Ensure that the center point of the protractor rests directly on the vertex of the angle.
-
Locate the appropriate scale: The protractor typically features two scales, one on the inner edge and one on the outer edge. Choose the scale that provides the most precise measurement for your angle.
-
Align the zero mark: Place the zero mark of the protractor on the initial arm of the angle. This will serve as your reference point.
-
Trace the other arm: Extend a ruler or pencil along the other arm of the angle. Follow this guiding line until it intersects with the inner scale of the protractor.
-
Read the measurement: Note the degree value where the extended line touches the inner scale. This value represents the measure of the angle.
-
Verify accuracy: Double-check the measurement by aligning the protractor with the second arm of the angle and ensuring that the zero mark aligns with this arm too. The reading should match your previous measurement, confirming the accuracy of your result.
Remember, precision is paramount when measuring angles. Align the protractor meticulously and read the scale with care. With these steps, you can harness the power of the protractor to unlock the secrets of angles.
Understanding Degrees and Minutes: The Building Blocks of Angle Measurement
Angles, the measure of the amount of turn a line makes, play a crucial role in geometry, engineering, and even everyday life. To understand angles, we need to delve into the world of degrees and minutes, the units that quantify their size.
Degrees and Minutes: The Angle Explorers
Imagine cutting a circle into 360 equal parts. Each part is known as a degree, and it represents one-three hundred and sixtieth of the circle. Degrees are the primary unit for angle measurement, denoted by the degree symbol (°).
But what if we need to measure angles that are smaller than one degree? Enter minutes. One minute is one-sixtieth of a degree, denoted by the prime symbol (‘). So, there are 60 minutes in one degree.
Expressing Angles with Degree-Minute Notation
Angles are expressed using a combination of degrees and minutes. For example, an angle of 30 degrees and 45 minutes is written as 30°45′.
- Whole degrees: Written as whole numbers, followed by the degree symbol (e.g., 30°)
- Minutes: Written after whole degrees, followed by the prime symbol (e.g., 45′)
Real-World Applications: When Degrees and Minutes Matter
Angle measurement finds practical application in numerous fields:
- Construction: Angles determine the slope of roofs, the layout of walls, and the alignment of beams.
- Engineering: Angles define the angles of joints, bridges, and aircraft wings.
- Navigation: Angles help sailors and pilots determine their direction and location.
- Healthcare: Angles are used in X-rays and MRIs to assess joint angles and measure bone alignment.
By understanding the concept of degrees and minutes, we can unlock the power of angle measurement, essential for comprehending the world around us.
Measuring Angle EFD with a Protractor: A Step-by-Step Guide
Measuring angles accurately is essential in various fields, from engineering to cartography. One indispensable tool for this task is the protractor, a device that helps us determine the measure of angles with precision.
Step 1: Preparing the Protractor
Before embarking on the measurement, place the protractor on a flat surface and ensure that its base is aligned with one side of the angle you wish to measure. Orient the protractor such that the zero degree mark coincides with the vertex of the angle, represented by E in the case of angle EFD.
Step 2: Determining the Angle Measure
Now, locate the point on the other side of the angle, labeled F in angle EFD. Trace an imaginary line from point F through the protractor’s center. Note the degree and minute markings where the line intersects the protractor’s scale. This value represents the measure of angle EFD.
Importance of Accurate Alignment
When using a protractor, meticulous alignment is paramount. The base of the protractor must be precisely parallel to the side of the angle, and the center should be perfectly superimposed over the angle’s vertex. Any slight misalignment will result in an erroneous measurement.
Example: Measuring Angle EFD
For instance, suppose we need to determine the measure of angle EFD. By following the steps outlined above, we carefully align the protractor and locate the point F on the opposite side of the angle. We then draw an imaginary line from F through the protractor’s center and observe that the line intersects the scale at 67 degrees and 30 minutes. Therefore, the measure of angle EFD is 67 degrees 30 minutes, or 67°30′.
By mastering the art of using a protractor, we empower ourselves to measure angles with accuracy and confidence, enabling us to solve problems and make informed decisions in fields where angular measurements play a crucial role.