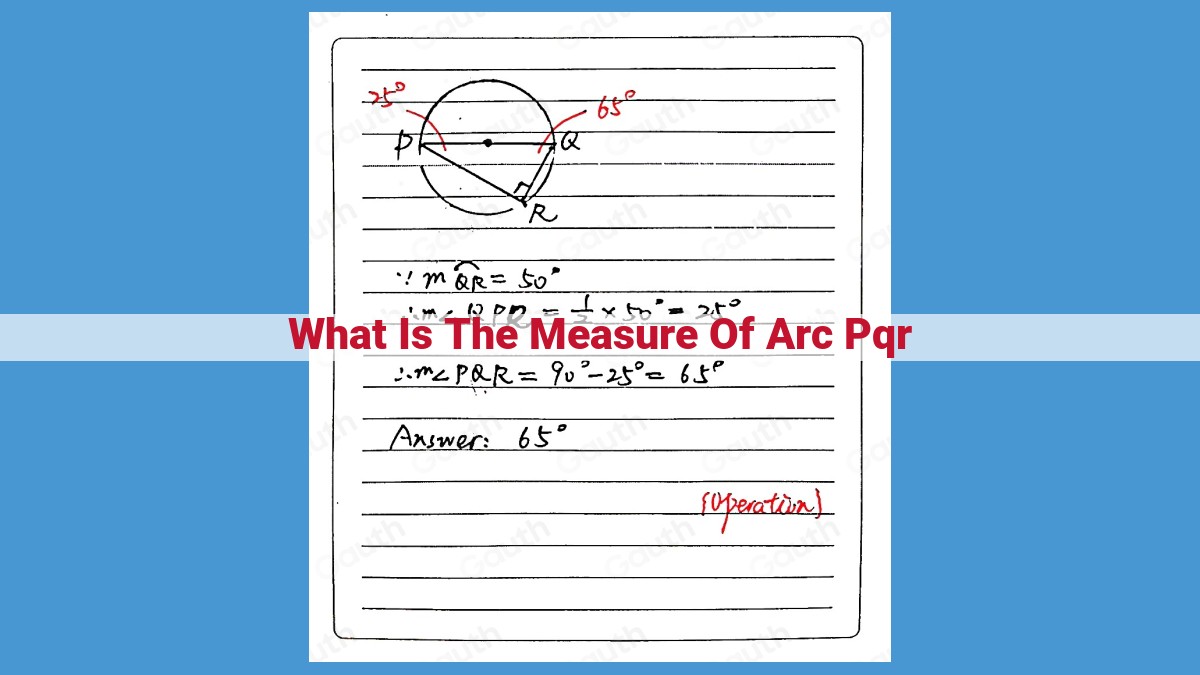
To determine the measure of arc PQR, you can calculate its degree measure or radian measure. Degree measure is defined using inscribed angles and is calculated by dividing the arc length by the circle’s circumference and multiplying by 360 degrees. Radian measure is defined based on the unit circle and is calculated by dividing the arc length by the radius. These measures provide a means to quantify the extent of an arc along the circumference of the circle.
Measuring Arcs: Unraveling the Secrets of Circular Geometry
In the fascinating world of geometry, arcs play a crucial role in defining and measuring circles. An arc is a portion of a circle’s circumference, connecting two points on the circle. It’s a fundamental element in understanding the geometry of circles and their properties.
Arcs can be measured in two primary ways: degree measure and radian measure. Both measures provide valuable insights into the size and extent of arcs, each with its unique advantages and applications.
The degree measure is based on the concept of inscribed angles. An inscribed angle is an angle formed by two radii drawn from the center of the circle to the endpoints of the arc. The degree measure of an arc is equal to the measure of its inscribed angle. This measure is commonly used in fields such as navigation and surveying.
On the other hand, the radian measure is based on the unit circle. The unit circle is a circle with a radius of 1. The radian measure of an arc is defined as the length of the arc on the unit circle. This measure is widely used in mathematics, physics, and engineering due to its unitless nature and its close relationship with the trigonometric functions.
Measuring Arcs: A Guide to Degree Measure
In the realm of geometry, arcs play a crucial role in understanding the properties of circles. Measuring these arcs accurately is essential for tackling various circular geometry problems. One widely used method for measuring arcs involves using degree measure.
Defining Degree Measure of an Arc
The degree measure of an arc is determined by the inscribed angle that intercepts it. This inscribed angle is formed by two radii drawn from the endpoints of the arc to the center of the circle. The degree measure of the arc is equal to the measure of its inscribed angle. In other words, it represents the central angle of the inscribed angle.
Formula for Degree Measure
The formula for calculating the degree measure of an arc is:
Degree Measure = (Central Angle Measure in Degrees) / 360 * 2πr
where:
- Degree Measure is the measure of the arc in degrees
- Central Angle Measure is the measure of the central angle in degrees
- r is the radius of the circle
Example Calculations
Let’s consider a few examples to illustrate the use of this formula:
- If an arc has a central angle measure of 60 degrees and the circle has a radius of 5 units, the degree measure of the arc is:
Degree Measure = (60°) / 360 * 2π(5) = 5π/3 ≈ 5.24 radians
- For an arc with a degree measure of 90 degrees and a circle with a radius of 7 units, we can find the central angle measure:
Central Angle Measure = Degree Measure * 360 / 2πr = 90° * 360° / 2π(7) ≈ 42.86°
By understanding the concept of degree measure, we can accurately determine the size of arcs and explore the fascinating world of circular geometry.
Radian Measure of Arc: Unraveling the Circular Mystery
In the realm of geometry, where circles reign supreme, understanding the intricacies of arcs is pivotal. Among the various ways to quantify arcs, the radian measure stands out as a fundamental concept. Let’s embark on a storytelling journey to unravel the secrets of radian measure.
The foundation of radian measure lies in the concept of the unit circle. Imagine a circle with a radius of 1 unit. When a central angle is formed, the length of the intercepted arc on the unit circle is the radian measure of the angle.
Calculating the Radian Measure
To determine the radian measure of an arc, we simply divide the arc length by the radius of the circle. The formula for radian measure can be expressed as:
Radian measure = Arc length / Radius
This formula highlights the direct relationship between the arc length and the radian measure. As the arc length increases, the radian measure also increases.
Unitless Nature and Representation
Unlike degree measure, which is measured in degrees, radian measure is unitless. This means it is independent of the specific unit of measurement used for the radius. Radian measure is represented by the symbol rad or simply left as a numerical value.
Examples: Radian Measure in Practice
Consider an arc of length 10 units on a circle with a radius of 5 units. Using the formula, we can calculate the radian measure as follows:
Radian measure = 10 units / 5 units = 2 rad
This indicates that the central angle associated with this arc has a radian measure of 2.
In another example, if the arc length is π units and the radius is 1 unit, the radian measure becomes:
Radian measure = π units / 1 unit = π rad
This result demonstrates the connection between the circumference of a circle and radian measure. A full circle, with an arc length equal to its circumference, has a radian measure of 2π rad.
The radian measure of an arc provides a versatile and unitless method of quantifying circular arcs. It plays a crucial role in understanding circular geometry and has applications in various fields, such as physics, engineering, and mathematics. Embracing the concept of radian measure will unlock a deeper appreciation for the intricacies of circular geometry and its significance in the realm of knowledge.
Determining the Length of an Arc: A Geometrical Excursion
In the realm of circular geometry, arcs hold immense significance. They represent segments of a circle’s circumference and serve as building blocks for understanding circular shapes and their properties. To fully grasp the nature of arcs, it’s essential to explore how we measure their length.
Unveiling the Formula
The formula for calculating the length of an arc is an elegant expression that intertwines the concepts of arc length, radius, and central angle. It states that:
Arc Length = Radius × Central Angle (in radians)
This formula provides a straightforward method for determining the distance along the arc, given the radius of the circle and the angle subtended by the arc at the circle’s center.
Understanding the Interplay
The formula highlights the close relationship between the arc’s length, the radius, and the central angle. As the radius increases, the arc length also increases, assuming the same central angle. Similarly, as the central angle gets larger, the arc length grows, while the radius remains constant.
Examples to Illuminate
To illustrate the formula’s application, let’s consider a few examples:
- Example 1: If a circle has a radius of 5 cm and the central angle measuring the arc is 60 degrees, which is pi/3 radians, the arc length is:
Arc Length = 5 cm × (pi/3 radians) ≈ 5.24 cm
- Example 2: Suppose an arc subtends an angle of 120 degrees, or 2pi/3 radians, at the center of a circle with a radius of 8 cm. The arc length is:
Arc Length = 8 cm × (2pi/3 radians) ≈ 16.96 cm
These examples showcase how the formula helps determine the length of an arc based on the given radius and central angle. By grasping the interplay between these variables, we unlock a deeper understanding of arc length and its applications in diverse areas of mathematics, engineering, and other fields.
Angle Formed by the Radius Drawn at Endpoints of Arc
In the world of circular geometry, we often encounter arcs, which are portions of circles. Measuring these arcs is crucial for understanding their properties and solving related problems. One way to measure arcs is by using inscribed angles.
An inscribed angle is an angle whose vertex lies on the circle and whose sides intersect the circle at two distinct points. The central angle of an arc is the angle formed by two radii drawn from the center of the circle to the endpoints of the arc. The inscribed angle is half the measure of its corresponding central angle. This means that if the central angle measures x degrees, then the inscribed angle will measure x/2 degrees.
This relationship between inscribed and central angles is a powerful tool for measuring arcs. By finding the inscribed angle, we can easily determine the degree measure of the arc. For example, if we have an arc with an inscribed angle of 60 degrees, we know that the corresponding central angle measures 120 degrees.
To summarize, the inscribed angle of an arc is an essential concept in circular geometry. It provides a straightforward way to determine the degree measure of the arc, making it an invaluable tool in solving various geometric problems.