The distribution coefficient, a measure of substance distribution between two immiscible phases, is crucial in understanding solute behavior in solvent extraction. It is the ratio of solute concentrations in the two phases at equilibrium. To calculate it, a sample is shaken in a separatory funnel to establish equilibrium, and solute concentrations in both phases are determined using methods like absorbance measurements. The distribution coefficient, often expressed as a partition coefficient, provides insights into solute solubility, drug absorption, pollutant behavior, and food analysis, making it a valuable tool in various fields.
Embarking on the Adventures of Distribution Coefficients: A Guide to Understanding Substance Behavior in Two-Phase Systems
In the realm of chemistry and beyond, substances often play hide-and-seek with their surroundings. Imagine a timid molecule hiding within a two-phase system, like a shy child seeking refuge in the shadows. Distribution coefficients are the secret maps that reveal the whereabouts of these elusive substances, shedding light on their behavior and guiding us towards a deeper understanding of the molecular world.
Distribution coefficients are the enigmatic guides that bridge the gap between two worlds, quantifying the preference of substances for one phase over another. When two immiscible liquids meet, like oil and water, they create a two-phase system where molecules face a choice: to mingle with the aqueous environment or embrace the non-polar embrace of the organic realm. Distribution coefficients unlock the secrets of this molecular decision-making process, revealing how substances interact with different solvents and environments.
Two-Phase System
- Explain the concept of a two-phase system, its relevance to distribution coefficient calculations, and the immiscibility of the aqueous and organic phases.
Two-Phase Systems: The Foundation of Distribution Coefficient
Picture this: you shake together a mixture of water and oil. What do you observe? Two distinct layers form, one on top of the other. This is a classic example of a two-phase system, a crucial concept in understanding the behavior of substances in different environments.
In the context of distribution coefficient calculations, we encounter two-phase systems where one phase is aqueous (water-based) and the other is organic (non-polar solvent-based). The immiscibility of these two phases means they do not dissolve into each other, creating a clear boundary between them.
The distribution coefficient, a measure of how a substance partitions between two immiscible phases, is heavily influenced by the nature of the two-phase system. It plays a vital role in predicting the fate and transport of solutes in various environmental and industrial processes.
Solvent Extraction
- Describe solvent extraction as a technique for separating components based on their different solubilities in two immiscible solvents. Explain the principle behind the transfer of solute from one phase to another.
Solvent Extraction: Unlocking Selective Separation with Immiscible Solutions
In the realm of chemistry, solvent extraction emerges as a powerful tool for separating components that play hide-and-seek within a two-phase system. Immiscible solvents, like water and oil, serve as the stage for this captivating dance of molecules.
Imagine a solute, a substance of interest, trapped within a two-phase system. Determined to liberate this captive, chemists employ a solvent with a strong affinity for the solute. This solvent, like an eager kidnapper, snatches the solute from its original solvent and transports it to its own domain, creating a new equilibrium.
This remarkable process, solvent extraction, hinges on the principle of selective solubility. The solvent chosen for the rescue mission must have a greater affinity for the solute than the solvent it currently resides in. This way, the solute is tempted to abandon its former home and join the new solvent.
As the extraction proceeds, the solute partitions between the two solvents, settling into a dynamic balance. This equilibrium is a delicate dance, where the concentration of the solute in each solvent remains constant over time. It’s a testament to the harmony that can be achieved between molecules.
The partition coefficient, a numerical dance partner, quantifies the affinity of the solute for each solvent. It’s a measure of how readily the solute switches allegiances, from one solvent to another. Factors like temperature, pH, and the solute’s own molecular characteristics influence this delicate dance.
In a separatory funnel, a sleek laboratory vessel, the two solvents engage in a gentle waltz. The extracted solute, now residing in its new solvent, separates from its former companion. This separation, a triumph of chemistry’s sleight of hand, allows scientists to isolate and analyze the solute, unlocking secrets and advancing our understanding of the molecular world.
The Partition Coefficient: Understanding the Distribution of Solutes
In the realm of chemistry, substances interact with their surroundings in complex ways. When two immiscible phases, such as water and an organic solvent, coexist, certain substances distribute themselves differently between them. This phenomenon is quantified by the partition coefficient, a crucial parameter that governs the behavior of solutes in such systems.
The partition coefficient, denoted as P, is defined as the ratio of solute concentration in one phase to that in the other, when the system has reached equilibrium. This ratio represents the solute’s relative affinity for each phase. Factors that influence the partition coefficient include the solute’s:
- Polarity: Polar solutes favor the aqueous phase, while nonpolar solutes prefer the organic phase.
- Molecular size and shape: Smaller, more compact molecules tend to have higher partition coefficients.
- Ionization state: Ionized solutes are less likely to partition into the organic phase because they cannot cross the nonpolar barrier.
- Temperature: Increasing temperature generally decreases the partition coefficient, making solutes more soluble in water.
- pH: pH can affect the ionization state of solutes, altering their partition coefficient.
Separatory Funnel: A Key Tool for Solvent Extraction
In the fascinating world of chemistry, we often encounter situations where we need to separate substances based on their different properties. One such technique that we employ is solvent extraction, where we use two immiscible solvents to coax a solute from one phase into the other. And in this process, the separatory funnel stands as an indispensable tool.
Imagine a clear, pear-shaped vessel with a narrow stem and a stopcock at the bottom – that’s a separatory funnel for you. This humble apparatus plays a pivotal role in separating the two solvents, allowing us to isolate and collect the extracted solute.
The separatory funnel works on the principle of gravity. We carefully introduce our mixture of two immiscible solvents into the funnel. As the liquids settle down, their immiscibility ensures that they form distinct layers. The denser solvent sinks to the bottom, while the less dense solvent floats on top.
Now comes the clever part. By carefully opening the stopcock, we can drain off the lower solvent, leaving the upper solvent and the extracted solute behind in the funnel. This simple yet effective process allows us to separate the two phases, paving the way for further analysis and purification of the desired compound.
The separatory funnel is a cornerstone in the chemist’s toolkit, enabling us to extract and isolate substances with precision and efficiency. It’s a versatile tool that finds applications in countless fields, from pharmaceutical research to environmental monitoring. So, when you’re next faced with the challenge of separating two immiscible solvents, reach for the trusty separatory funnel – it will guide you to a successful extraction every time.
Concentration
- Define concentration and discuss its significance in distribution coefficient calculation. Explain how concentration is expressed in different units such as molarity, molality, or mass fraction.
Concentration: The Essential Ingredient for Understanding Distribution Coefficients
When it comes to understanding how substances behave in a two-phase system, the distribution coefficient plays a crucial role. The distribution coefficient measures the relative distribution of a substance between two immiscible phases. To calculate the distribution coefficient accurately, we need to consider the concentration of the substance in each phase.
Concentration refers to the amount of substance present in a given volume of solvent. It can be expressed in various units, each with its own significance. One commonly used unit is molarity, which represents the number of moles of solute per liter of solution. Another unit is molality, which expresses the number of moles of solute per kilogram of solvent. Mass fraction, on the other hand, represents the mass of solute per total mass of the solution.
The choice of concentration unit depends on the specific application. In distribution coefficient calculations, molarity is often preferred because it allows for direct comparisons of solute concentrations in different solvents. It simplifies the calculation process and provides a clear understanding of the substance’s distribution.
By determining the concentration of a substance in each phase at equilibrium, we can accurately calculate the distribution coefficient. The distribution coefficient provides valuable insights into the behavior of the substance in the two-phase system, allowing us to make informed decisions and predictions in various fields of science and industry.
Equilibrium and Distribution Coefficient Determination
In the realm of chemistry, equilibrium is a pivotal concept that plays a central role in understanding and predicting the behavior of substances in various systems. In the context of distribution coefficient determination, equilibrium holds particular significance.
What is Equilibrium?
Equilibrium is a state in which opposing forces or tendencies cancel each other out, resulting in no net change over time. In the case of distribution coefficient determination, equilibrium is reached when the distribution ratio, which is the ratio of solute concentrations in the two phases at equilibrium, remains constant.
Achieving Equilibrium
Reaching equilibrium is a crucial step in determining the distribution coefficient accurately. To achieve equilibrium, sufficient time must be allowed for the solute to distribute itself between the two phases until the distribution ratio no longer changes. This can be experimentally determined by repeatedly measuring the distribution ratio until a consistent value is obtained.
Importance of Equilibrium
Equilibrium is essential for distribution coefficient determination because it ensures that the distribution of the solute between the two phases is representative of the true equilibrium state. If equilibrium is not reached, the distribution ratio may not accurately reflect the solute’s behavior in the system.
Monitoring Equilibrium
To monitor equilibrium, the distribution ratio can be measured repeatedly over time. If the distribution ratio changes, it indicates that equilibrium has not yet been achieved. The measurements should continue until a constant distribution ratio is observed, indicating that equilibrium has been established.
Consequences of Non-Equilibrium
If distribution coefficient determination is performed in the absence of equilibrium, the results may be inaccurate and unreliable. This can lead to incorrect predictions about the behavior of the solute in the system and hinder the effective application of the distribution coefficient in various fields.
Distribution Ratio
- Define the distribution ratio as the ratio of solute concentrations in the two phases at equilibrium. Explain its relationship to the partition coefficient and concentration.
Distribution Ratio: A Measure of Solute Partitioning in Two-Phase Systems
The distribution ratio is a crucial parameter that quantifies the distribution of a solute between two immiscible phases, such as an aqueous phase and an organic phase. It plays a significant role in understanding the behavior of substances in a two-phase system and finds applications in various scientific fields.
The distribution ratio (D) is defined as the ratio of the equilibrium concentrations of a solute in the two phases. It is expressed as:
_D_ = [Solute] in organic phase / [Solute] in aqueous phase
where [Solute] represents the concentration of the solute in the respective phase.
The distribution ratio is closely related to the partition coefficient (P) of the solute, which is the ratio of its solubilities in the two phases. Mathematically, D is equal to P multiplied by the ratio of the volumes of the organic and aqueous phases.
The value of D provides insights into the solute’s preference for one phase over the other. A high D indicates that the solute is more soluble in the organic phase, while a low D suggests that it is more soluble in the aqueous phase. Factors such as the solute’s polarity, structure, and the nature of the solvents influence its distribution ratio.
In solvent extraction, the distribution ratio is used to determine the efficiency of the extraction process. A higher D indicates a more efficient extraction, as the solute is preferentially partitioned into the organic phase. Analytical techniques, such as spectrophotometry or chromatography, are employed to measure solute concentrations in each phase and calculate the distribution ratio.
The distribution ratio has practical applications in fields such as pharmaceutical science, environmental chemistry, and food analysis. In pharmaceutical science, it helps predict drug absorption and distribution within the body. In environmental chemistry, it is used to study the behavior of pollutants in different environmental compartments, such as water and soil. In food analysis, it aids in determining the distribution of nutrients and contaminants in food systems.
Understanding the Distribution Ratio: A Practical Example
Imagine you have a mixture of a dye dissolved in water. You add this mixture to a separatory funnel and shake it with an immiscible organic solvent, such as dichloromethane. After shaking, the mixture separates into two layers: the aqueous phase (water) and the organic phase (dichloromethane).
You collect aliquots from both layers and measure the absorbance of the dye in each phase using a spectrophotometer. The absorbance is directly proportional to the concentration of the dye. Let’s say you obtain an absorbance of 0.5 in the aqueous phase and 1.0 in the organic phase.
The distribution ratio (D) in this case would be:
_D_ = [Dye] in organic phase / [Dye] in aqueous phase
= 1.0 / 0.5
= 2
This D value of 2 indicates that the dye has a preference for the organic phase, as it is twice as concentrated in that phase compared to the aqueous phase. This information can be useful in optimizing the extraction process for the desired compound.
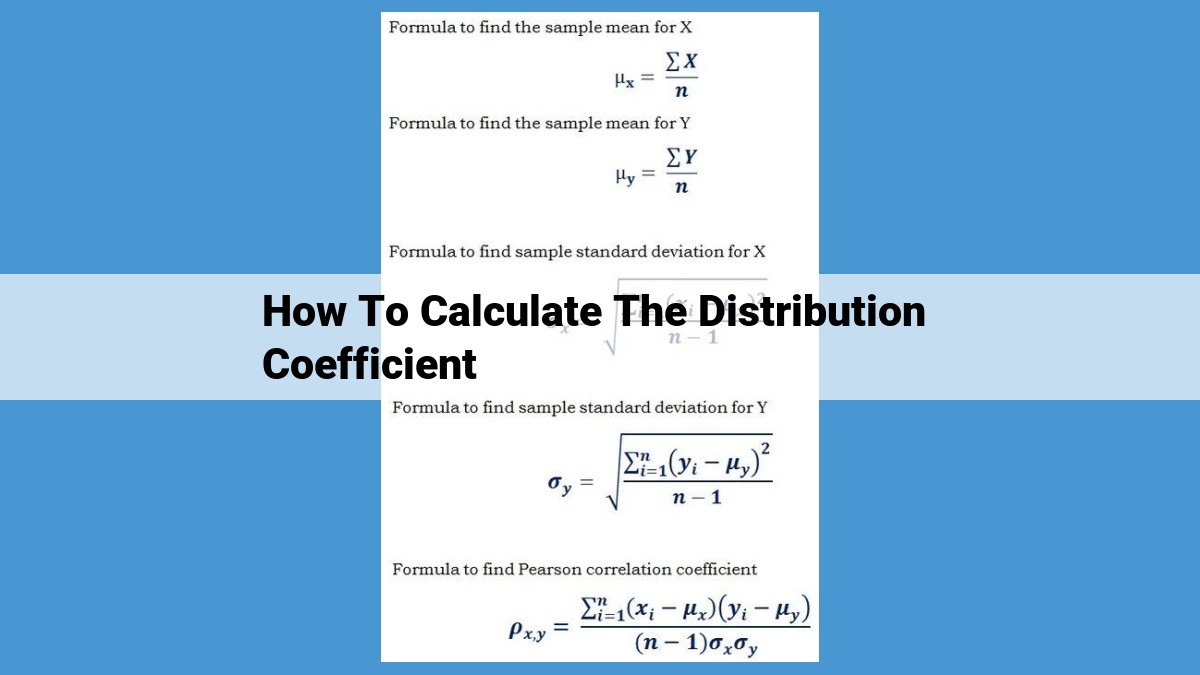
Calculating the Distribution Coefficient: A Step-by-Step Guide
In the realm of chemistry, the distribution coefficient plays a pivotal role in comprehending the behavior of substances within a two-phase system. This coefficient quantifies the extent to which a solute partitions between two immiscible phases, providing valuable insights into its solubility and partitioning preferences.
Step 1: Determine the Distribution Ratio
The distribution ratio serves as the foundation for calculating the distribution coefficient. It represents the ratio of solute concentrations in the two phases at equilibrium. To determine the distribution ratio experimentally, scientists employ various analytical techniques, such as spectrophotometry, to measure the concentration of the solute in each phase.
Step 2: Calculate the Concentration of the Solute
Once the distribution ratio is obtained, the next step involves calculating the concentration of the solute in each phase. This concentration can be expressed in different units, including molarity, molality, or mass fraction. The choice of unit depends on the specific application and the desired level of accuracy.
Step 3: Determine the Equilibrium State
Equilibrium is crucial for accurate distribution coefficient determination. It signifies the point where the distribution ratio remains constant over time. To ensure that equilibrium has been reached, scientists typically allow sufficient time for the system to stabilize before taking measurements.
Step 4: Calculate the Distribution Coefficient
Finally, the distribution coefficient is calculated by dividing the concentration of the solute in one phase by its concentration in the other phase. This coefficient provides a quantitative measure of the solute’s preference for one phase over the other.
Examples and Applications
The distribution coefficient finds widespread application in diverse scientific disciplines, including:
- Pharmaceutical science: Predicting drug absorption and distribution within the body
- Environmental chemistry: Studying the behavior and fate of pollutants in different environmental compartments
- Food analysis: Determining the distribution of nutrients and contaminants in food systems
Applications
- Describe the practical applications of the distribution coefficient in various fields, such as:
- Pharmaceutical science (predicting drug absorption and distribution)
- Environmental chemistry (studying pollutant behavior in different environmental compartments)
- Food analysis (determining the distribution of nutrients and contaminants in food systems)
Applications of the Distribution Coefficient
The distribution coefficient, a crucial parameter in understanding the behavior of substances in a two-phase system, finds widespread applications across diverse fields.
In the pharmaceutical industry, it plays a pivotal role in predicting drug absorption and distribution in biological systems. By determining the partitioning tendency of a drug between blood plasma and different tissues, scientists can assess its therapeutic efficacy and potential side effects.
In environmental chemistry, the distribution coefficient is indispensable for studying the fate and transport of pollutants in the environment. By investigating how contaminants distribute between water, soil, and air, researchers can understand their mobility, persistence, and potential risks to ecosystems.
In food analysis, the distribution coefficient aids in determining the distribution of nutrients and contaminants in food products. Understanding how these substances partition between food components, such as fat and water, helps ensure food quality, optimize nutritional value, and identify potential hazards.
Moreover, the distribution coefficient also finds applications in:
- Chemical engineering: Designing separation processes, such as solvent extraction and chromatography.
- Materials science: Understanding the distribution of molecules within various materials and optimizing their properties.
- Biochemistry: Investigating the interactions of molecules with biological membranes and predicting membrane permeability.