To calculate transmittance from absorbance, use the formula T = 10^(-A), where T is transmittance and A is absorbance. Absorbance is the negative logarithm of transmittance, so by rearranging this equation, we can determine transmittance from absorbance. This calculation is useful in spectroscopy to quantify the amount of light transmitted through a sample and determine its concentration or other properties based on the Beer-Lambert Law, which relates absorbance to the sample’s concentration, path length, and molar absorptivity.
Unveiling the Secrets of Light and Matter: Transmittance and Absorbance in Spectroscopy
In the realm of science, where the wonders of the unseen world unfold, spectroscopy emerges as an indispensable tool. This powerful technique allows us to probe the interactions between light and matter, unlocking a wealth of secrets about the materials around us. Among the key concepts that underpin spectroscopy are transmittance and absorbance, two pivotal parameters that quantify the journey of light as it encounters various substances.
Transmittance: Unveiling the Light that Passes Through
Imagine a beam of light traveling through a material, like a ray of sunshine piercing through a windowpane. Some of that light finds its way through unscathed, while a portion of it gets absorbed by the material. Transmittance, denoted by the symbol T, measures the fraction of light that successfully navigates through the material. It represents the amount of light that emerges on the other side, relative to the initial intensity of the beam.
Absorbance: Quantifying the Light that’s Captured
In contrast to transmittance, absorbance, symbolized by A, measures the amount of light that a material absorbs. It represents the fraction of light that is intercepted and converted into other forms of energy, such as heat or chemical energy. Absorbance is inversely related to transmittance, meaning that as transmittance increases, absorbance decreases, and vice versa.
The Interplay of Transmittance and Absorbance
Transmittance and absorbance are two sides of the same coin, providing complementary information about the interaction of light with matter. The relationship between these two parameters is expressed by the following formula:
A = -log₁₀(T)
Where:
- A = Absorbance
- T = Transmittance
This formula demonstrates that the absorbance is the negative logarithm of the transmittance. In essence, it means that as transmittance decreases, absorbance increases, and vice versa.
Significance in Spectroscopy
The concepts of transmittance and absorbance are fundamental to the field of spectroscopy. By measuring these parameters, spectroscopists can gain insights into various characteristics of the sample under investigation, such as its concentration, composition, and molecular structure. These measurements form the basis of numerous analytical techniques, enabling the identification and quantification of substances in a wide range of applications, from environmental monitoring to medical diagnostics.
Understanding the Interplay between Transmittance and Absorbance
In the realm of spectroscopy, two fundamental concepts that govern the interaction of light with matter are transmittance (T) and absorbance (A). Transmittance measures the proportion of light that passes through a sample, while absorbance quantifies the amount of light absorbed by the sample.
The relationship between transmittance and absorbance is logarithmic and can be expressed as A = -log10(T). This formula reveals that as the transmittance decreases (indicating less light passing through the sample), the absorbance increases accordingly.
To understand this relationship, let’s consider the following scenario. Imagine shining a beam of light through a clear glass solution. If all the light passes through without being absorbed, the transmittance is 100% (T = 1.00) and the absorbance is 0. However, if all the light is absorbed by the solution, the transmittance becomes 0% (T = 0.00) and the absorbance reaches its maximum value. This maximum absorbance is a characteristic of the sample and its concentration.
The Beer-Lambert Law: Unraveling the Secrets of Light Absorption
Imagine you have a solution of an intriguing substance, and you’re eager to determine its concentration. You turn to the trusty technique of spectroscopy, which involves shining light through the solution and measuring how much light is absorbed.
This is where transmittance and absorbance come into play. Transmittance measures the fraction of light that passes through the solution, while absorbance measures the fraction that is absorbed. The two are inversely proportional, meaning higher absorbance indicates lower transmittance.
The Beer-Lambert Law is the fundamental equation that governs the relationship between absorbance, transmittance, and the properties of the solution. It states that:
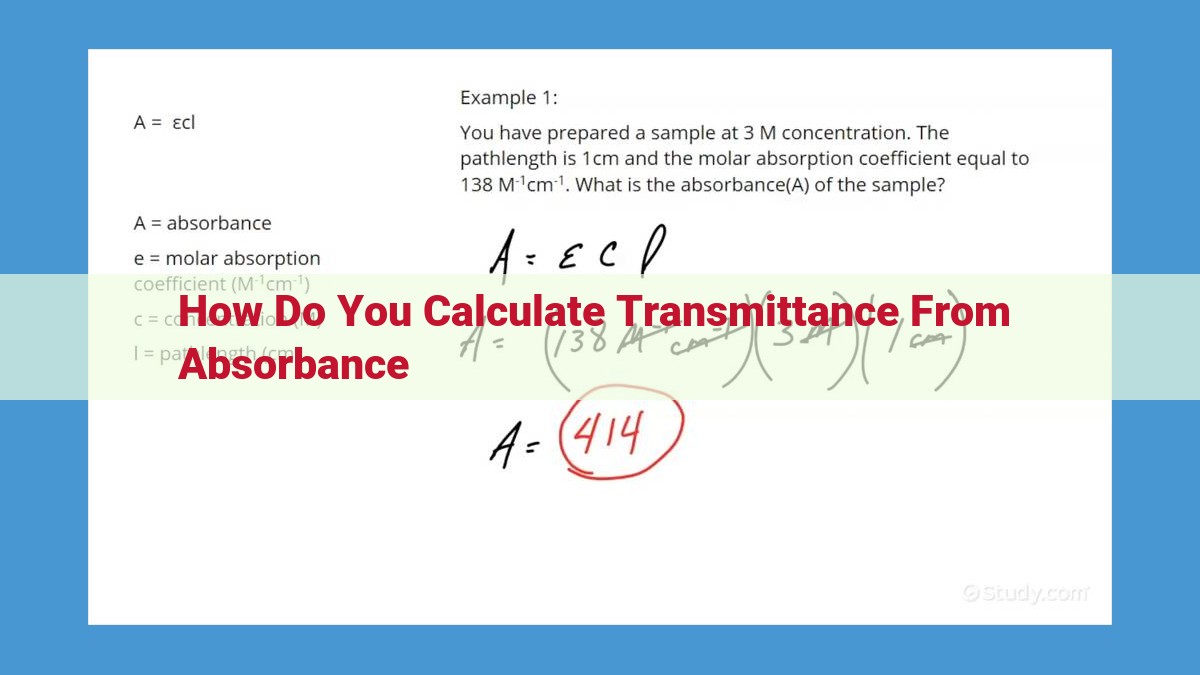
A = εbc
where:
- A is the absorbance
- ε is the molar absorptivity (a constant for a specific substance and wavelength)
- b is the path length (the distance the light travels through the solution)
- c is the concentration of the substance
The molar absorptivity is a characteristic property of the substance being measured. It represents the ability of the substance to absorb light at a specific wavelength. Path length is simply the thickness of the solution through which the light passes. Concentration is the amount of substance present in a given volume of solution.
So, how does this equation help us determine concentration? By rearranging it, we get:
c = A/(εb)
This formula allows us to calculate the concentration of the substance in the solution using measured absorbance values.
It’s important to note that the Beer-Lambert Law is only valid within a certain range of concentrations. Beyond this range, the relationship between absorbance and concentration becomes non-linear. Additionally, scattering of light and other factors can sometimes affect the accuracy of measurements.
Calculating Transmittance from Absorbance
Deriving the Formula
Transmittance and absorbance are intimately intertwined, and the Beer-Lambert Law provides a bridge between them. The Beer-Lambert Law states that:
A = εbc
where A is absorbance, ε is molar absorptivity, b is path length, and c is concentration.
To calculate transmittance (T) from absorbance, we rearrange the Beer-Lambert Law to solve for T:
T = 10^(-A)
Step-by-Step Guide to Using the Formula
-
Determine the Absorbance Value: Measure or obtain the absorbance value (A) from a spectrophotometer or other instrument.
-
Substitute A into the Formula: Plug the absorbance value into the formula T = 10^(-A). For example, if A = 0.5, then T = 10^(-0.5) = 0.316.
-
Calculate Transmittance: The result of the calculation is the transmittance value (T), which represents the fraction of light that passes through the sample without being absorbed.
Calculating transmittance from absorbance is a fundamental technique in spectroscopy. By understanding the relationship between these two parameters, we can analyze light-matter interactions and determine the concentration of a substance in a solution.
Additional Considerations
The Linear Range of the Beer-Lambert Law
The Beer-Lambert Law holds true only within a specific range of absorbance values, known as the linear range. Using absorbance values outside this range can lead to inaccurate measurements. This is because the relationship between absorbance and concentration becomes non-linear at higher absorbance values. Therefore, it’s crucial to determine the linear range for your specific system to ensure reliable results.
Scattering and Non-Linearity
In certain situations, factors such as scattering can affect the accuracy of absorbance measurements. Scattering occurs when light is deflected from its path due to particles or imperfections in the sample. This can result in reduced transmittance and increased absorbance, leading to potential errors in concentration determination.
Additionally, non-linearity can occur when the sample exhibits complex interactions with light. This can arise from phenomena such as fluorescence or energy transfer within the sample. In such cases, the relationship between absorbance and concentration may deviate from the Beer-Lambert Law, making it necessary to employ more sophisticated analytical techniques to obtain accurate results.