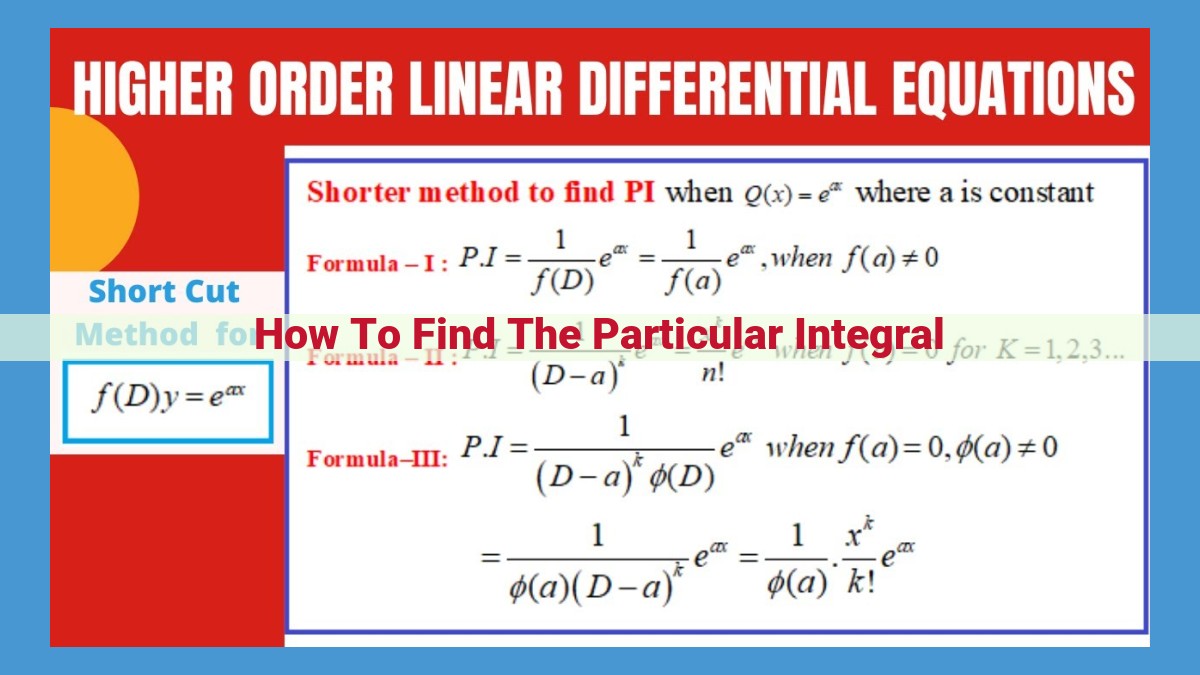
A particular integral is a solution to a differential equation that satisfies a specific forcing function. To find a particular integral, the method of undetermined coefficients or variation of parameters can be used. The method of undetermined coefficients is suitable for polynomial, exponential, or sinusoidal forcing functions, while the method of variation of parameters is more general. The superposition principle can be applied to combine particular integrals for multiple forcing functions. The existence of a particular integral is ensured if the constant term of the forcing function is zero.
- Definition of a particular integral
- Purpose of finding particular integrals
In the realm of Calculus and Differential Equations, we unravel the mystery of particular integrals. These elusive functions serve as the linchpin in understanding the behavior of nonhomogeneous differential equations that arise from real-world phenomena.
Definition and Purpose of Particular Integrals
A particular integral is the unique solution to a nonhomogeneous differential equation. It represents a function that, when substituted into the equation, yields a zero on the right-hand side. Finding particular integrals is vital because it allows us to model the specific response of a system to external forces.
Consider a differential equation like:
y'' + 2y' + y = sin(x)
The homogeneous part of this equation (without the sin(x) term) has solutions that describe the system’s natural oscillations. The particular integral, on the other hand, captures the system’s forced response to the sinusoidal input.
General Method for Finding Particular Integrals
- Method of undetermined coefficients
- Method of variation of parameters
Part 1: Understanding Particular Integrals
In the realm of mathematics, we encounter equations that describe the behavior of physical systems over time. Differential equations are especially powerful tools for unraveling the dynamic behavior of such systems. Integral equations, on the other hand, provide a method for finding solutions to differential equations.
Enter the concept of particular integrals, which represent specific solutions to differential equations that satisfy given conditions. They play a crucial role in understanding the response of a system to external influences or forcing functions.
Part 2: General Methods for Finding Particular Integrals
In the quest to determine particular integrals, we have at our disposal two primary methods:
1. Method of Undetermined Coefficients:
This method shines when the forcing function is a polynomial, exponential, or sinusoid. It involves guessing a particular integral with undetermined coefficients and then solving for those coefficients to match the forcing function.
2. Method of Variation of Parameters:
This method proves useful when the forcing function is more complex. It employs a system of differential equations to derive the particular integral as a product of the solution to the corresponding homogeneous equation and functions known as variation parameters.
Part 3: Choosing the Right Method
The suitability of each method depends on the nature of the forcing function. For instance, if the forcing function is a polynomial of degree n, the method of undetermined coefficients can yield a particular integral of the same degree n. On the other hand, the method of variation of parameters offers versatility in handling more intricate forcing functions.
Part 4: The Superposition Principle
A remarkable property of particular integrals is the superposition principle. When a system is subject to multiple forcing functions, we can determine the particular integral for each forcing function independently. The total particular integral is simply the sum of these individual integrals.
Part 5: Existence of Particular Integrals
While the methods described above are powerful, there exists a fundamental restriction on the existence of particular integrals. For a particular integral to exist, the forcing function must not contain a constant term. If it does, the corresponding differential equation may not possess a particular integral, indicating that the system cannot sustain a constant external influence.
Method of Undetermined Coefficients
- Used for polynomial, exponential, or sinusoidal forcing functions
- How to determine unknown coefficients
The Art of Determining Particular Integrals: Unveiling the Method of Undetermined Coefficients
In the realm of differential equations, particular integrals play a pivotal role in solving nonhomogeneous equations. They represent the unique solutions that cater to specific forcing functions, paving the way for an in-depth understanding of the equation’s behavior.
The method of undetermined coefficients emerges as a powerful tool in this pursuit, particularly when confronted with polynomial, exponential, or sinusoidal forcing functions. Its essence lies in making educated guesses for the form of the particular integral based on the nature of the forcing function.
Imagine an equation of the form:
a(x)y'' + b(x)y' + c(x)y = f(x)
where f(x) represents the forcing function. By assuming a particular integral of the form:
y_p(x) = Ax^n + Bx^(n-1) + ... + Cx^2 + Dx + E
where n is the degree of the forcing function and A, B, …, E are unknown coefficients, we embark on a quest to determine their values.
The key to this method lies in substituting the trial solution into the differential equation and equating coefficients of like terms on both sides of the equation. This leads to a system of algebraic equations that can be solved for the unknown coefficients.
For instance, consider an equation with a forcing function f(x) = x^2. Our trial solution would be:
y_p(x) = Ax^2 + Bx + C
Substituting into the equation and equating coefficients, we obtain:
2A + 0B + 0C = 0
0A + 2B + 0C = 0
0A + 0B + C = 1
Solving this system, we find A = 0.5, B = 0, and C = 1.
Thus, our particular integral is:
y_p(x) = 0.5x^2 + x
Armed with the method of undetermined coefficients, we gain the ability to conquer a vast spectrum of differential equations with nonhomogeneous terms. It provides a systematic and straightforward approach to finding particular integrals, empowering us to unlock the secrets of these complex equations.
The Method of Variation of Parameters: Unlocking Complex Particular Integrals
In the realm of differential equations, finding particular integrals plays a crucial role in understanding how systems respond to external influences. When dealing with more complex forcing functions that don’t neatly fit into polynomial, exponential, or sinusoidal categories, we turn to the Method of Variation of Parameters.
This method is a powerful tool that allows us to determine particular integrals for any forcing function. Unlike the Method of Undetermined Coefficients, which assumes a specific functional form for the particular integral, Variation of Parameters treats it as an unknown function.
Imagine a differential equation where the forcing function is an erratic, ever-changing entity. The Method of Variation of Parameters allows us to represent the particular integral as a superposition of unknown functions multiplied by the solution to the homogeneous equation. These unknown functions are then cleverly determined by solving a system of differential equations.
By tailoring the unknown functions to the forcing function, we ensure that the particular integral captures the nuances of the external influence. It’s like fitting a custom-made key to unlock the intricacies of the differential equation.
While the Method of Variation of Parameters involves more complex calculations than the Method of Undetermined Coefficients, it extends our ability to find particular integrals to a much wider range of problems. It empowers us to tackle differential equations that describe real-world phenomena where forcing functions are often far from simple.
Particular Integrals and the Superposition Principle
Discovering Particular Integrals
In the realm of differential equations, particular integrals play a crucial role in addressing specific scenarios involving non-homogeneous equations. These equations arise when they are influenced by external forces, known as forcing functions. Particular integrals represent solutions tailored to these specific forcing functions.
The General Approach
To determine particular integrals, we leverage two primary methods: the method of undetermined coefficients and the method of variation of parameters. The former proves effective for polynomial, exponential, or sinusoidal forcing functions, while the latter handles more general scenarios.
Combining Forces with Superposition
When multiple forcing functions act simultaneously, the superposition principle comes into play. This principle asserts that the particular integral for the combined forcing functions is simply the sum of the particular integrals for each individual forcing function.
A Real-World Analogy
Imagine a ship sailing through the ocean, encountering waves from various directions. Each wave can be viewed as a forcing function. The principle of superposition suggests that the ship’s overall motion is merely the sum of its responses to each individual wave.
Particular integrals and the superposition principle are indispensable tools in solving differential equations with non-homogeneous terms. By utilizing these techniques, we can effectively address a wide range of real-world scenarios where external forces influence system behavior.
Finding Particular Integrals: The Key to Solving Differential Equations with Forcing Functions
In the realm of differential equations, finding particular integrals plays a crucial role in solving equations with external forcing functions. A particular integral represents a solution to an equation that satisfies the forcing function’s specific requirements.
The Method of Undetermined Coefficients: A Straightforward Approach
The method of undetermined coefficients shines when dealing with polynomial, exponential, or sinusoidal forcing functions. By assuming a solution that mimics the forcing function’s form and then determining unknown coefficients, we can find the particular integral. This method simplifies the process for common forcing functions.
The Method of Variation of Parameters: A More Versatile Option
For more general forcing functions, the method of variation of parameters proves more effective. This technique involves solving a system of differential equations to determine the unknown coefficients in our solution. It provides a broader range of options for solving particular integrals.
The Superposition Principle: Combining Solutions
The superposition principle offers a convenient way to handle multiple forcing functions. It states that the particular integral for a linear combination of forcing functions is simply the sum of the particular integrals for each individual forcing function. This principle helps us efficiently solve complex problems.
The Existence of Particular Integrals: A Crucial Consideration
However, the existence of a particular integral requires a specific condition to be met: the constant term in the forcing function must be zero. This condition ensures that the particular integral doesn’t contain a term that grows unboundedly over time. If this condition isn’t satisfied, finding a particular integral may not be possible.
By mastering these techniques, we gain the ability to solve a wide range of differential equations with forcing functions. These equations play a vital role in modeling physical phenomena and engineering applications, making the study of particular integrals essential for engineers, scientists, and mathematicians alike.