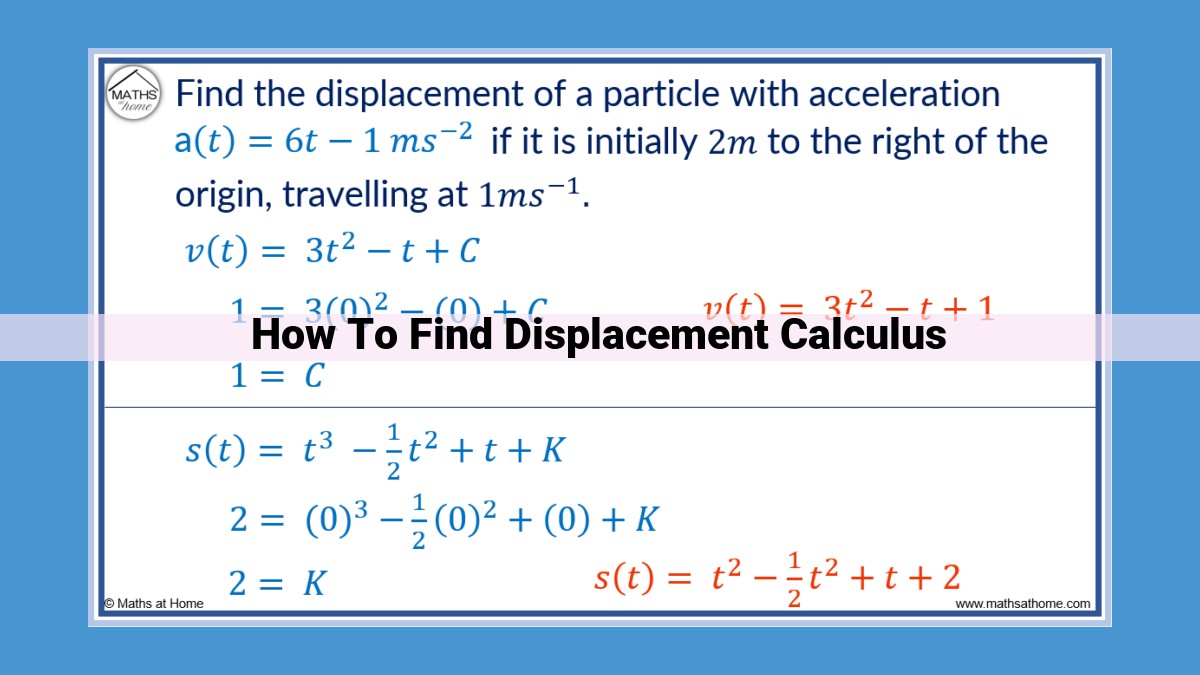
Displacement, a crucial concept in calculus, measures an object’s position change. To find displacement graphically, the area under the velocity-time curve represents the total displacement. Alternatively, the initial position can be subtracted from the final position, which is the position at a given time, found by integrating the velocity function. Understanding the sign of displacement indicates the direction of movement. Calculus plays a vital role in displacement calculations, as the velocity function, the derivative of the position function, provides valuable information about an object’s motion.
How to Find Displacement Using Calculus: A Journey Through Time and Space
In the realm of physics, displacement reigns supreme. It’s the quintessential measure of an object’s change in position, a testament to its journey through time and space. But how do we uncover this elusive quantity? Calculus, the wizard of motion and change, holds the key.
Displacement: The Essence of Motion
Imagine a ball soaring through the air. As it arcs and descends, its position shifts. Displacement, like a watchful guardian, captures this change. It’s the difference between the ball’s initial and final positions, a geometric representation of its movement.
Calculus: The Guide to Displacement’s Secrets
Calculus empowers us to unravel displacement’s intricate dance. By introducing the area under a velocity-time graph, we unlock a hidden treasure. This area, like a flowing tapestry, tells the tale of the object’s displacement.
Velocity, the rate at which the ball moves, weaves this tapestry. Graphically, it’s a curve that charts the ball’s speed over time. Calculus reveals that the integral of velocity, like the summation of these tiny speed segments, calculates the displacement.
Distance vs. Displacement: Unveiling the Distinction
Beware, dear reader, for distance and displacement are often confused. Distance, the total length of the ball’s path, can be likened to the winding road it travels. Displacement, on the other hand, is the straight-line distance between the ball’s starting and ending points.
Establishing a Reference Point: The Anchor of Displacement
To embark on our displacement quest, we must establish an initial position, a point of reference from which we measure change. This reference point, like a lighthouse in the stormy sea of motion, provides a stable foundation for our calculations.
Position Function: Time’s Tale of Location
The position function, a mathematical storyteller, chronicles the ball’s position at any given moment. It’s a time-based function that paints a vivid picture of the ball’s movements.
Sign of Displacement: A Directional Compass
Displacement, like a two-faced enigma, can be positive or negative. This sign, like a compass needle, points towards the direction of the object’s movement. Positive displacement denotes a journey in one direction, while negative displacement signals a shift in the opposite direction.
Velocity Function: The Messenger of Change
The velocity function, the derivative of the position function, unveils the ball’s instantaneous velocity. It’s a dynamic force that captures the rate at which the ball’s position changes.
Area Under the Velocity Curve: A Graphical Representation of Displacement
Imagine a car speeding down a highway, weaving in and out of traffic. Its velocity, a measure of its instantaneous speed and direction, is constantly changing. But amid these fluctuations, its displacement, or the net change in its position relative to a fixed point, is a more stable quantity.
The area under the velocity-time graph for this car provides a graphical representation of its displacement. This area is like a visual record of the car’s total distance traveled, both forward and backward, at any given moment.
Instantaneous velocity, represented by the slope of the velocity-time graph at a given point, measures the car’s speed and direction at that instant. Average velocity, on the other hand, is the slope of the line connecting two points on the graph, indicating the overall speed and direction of the car over that time interval.
The integral is a mathematical tool that allows us to calculate the area under the velocity-time graph. This integral represents the total displacement of the car over the time interval covered by the graph. In essence, it provides a numerical value for the area bounded by the velocity curve, the horizontal axis, and the two vertical lines marking the start and end of the time interval.
By understanding the concept of area under the velocity curve, we gain a deeper insight into displacement and its relationship to velocity. This knowledge is essential in various fields, including physics, engineering, and computer graphics, where accurate calculation of displacement is crucial.
Distance vs. Displacement: Unraveling the Distinction
In the realm of physics, two closely related yet distinct concepts often confound students and enthusiasts alike: distance and displacement. While they share certain similarities, their fundamental differences can lead to confusion if not properly understood.
Distance represents the total length of the path traveled by an object as it moves from one point to another. It is a scalar quantity, meaning it has only magnitude and no direction. Imagine a car driving along a winding road; the distance traveled would be the sum of all the curves and bends it navigates.
Displacement, on the other hand, is the straight-line distance between the object’s initial and final positions. Unlike distance, displacement is a vector quantity, meaning it has both magnitude and direction. In our car analogy, the displacement would be the direct line drawn from the car’s starting point to its ending point, regardless of the actual path taken.
The crucial distinction between distance and displacement lies in the notion of path. Distance considers the entire trajectory, while displacement focuses solely on the net change in position. In other words, distance accounts for every twist and turn, while displacement represents the overall progress made towards a final destination.
Understanding this difference is essential in various applications of physics, such as mechanics, kinematics, and engineering. It allows us to accurately quantify the motion of objects and determine their trajectory and overall progress.
Initial Position: The Foundation for Displacement
In our quest to understand displacement, the initial position plays a pivotal role. This critical value establishes the starting point of an object’s journey, providing a reference against which we can measure its change in position.
Just as in a race, we need to know where the runners start from to determine who has traveled the farthest, the initial position serves as the baseline for displacement. This is especially crucial when dealing with complex motions where the starting point may not be obvious.
Choosing a suitable reference point is an essential part of defining the initial position. This reference point does not have to be the physical location of the object; it can be any convenient point from which to measure displacement. For example, in the case of a moving car, the reference point could be the roadside or a specific landmark. By establishing a clear reference point, we can accurately determine the object’s displacement relative to that point.
Unlocking the Secrets of Displacement: A Calculus-Based Approach
In the realm of physics, understanding displacement is paramount. It’s the key to comprehending how objects move and change position over time. And when it comes to calculating displacement, calculus steps in as an indispensable tool.
The Dance of Calculus and Displacement
Imagine an object zipping along a path. As it moves, its velocity, or rate of change in position, fluctuates. Calculus provides a way to capture this intricate dance by revealing the relationship between velocity and displacement.
The position function is a magical function that holds the secret to an object’s position at any given moment. It’s like a time-lapse camera, capturing the object’s journey frame by frame. And guess what? The velocity function is the position function’s superhero sidekick, always one step ahead, predicting how the object’s position will change in the next instant.
Connecting the Dots: Calculus Unveils the Truth
The beauty of calculus shines through when we connect these two functions. The derivative of the position function, ladies and gentlemen, is none other than the velocity function! This powerful mathematical operation unveils the intricate dance between position and velocity.
Just as a velocity graph captures the object’s speed and direction, the position function tells us where it is at any given time. By mastering this mathematical connection, we gain the power to calculate displacement, the ultimate measure of how far and in which direction an object has moved.
The Sign of Displacement: Your Compass for Directionality
In our journey to understand displacement using calculus, the sign of displacement emerges as a crucial indicator of directionality. Displacement, as we know, represents the change in position of an object. And just like any change, it can be positive or negative, depending on the object’s motion.
When displacement is positive, it signifies that the object has moved in the positive direction. This direction is often defined with respect to a reference point. For instance, if we consider the positive direction to be rightward, then a positive displacement indicates the object has moved to the right. Conversely, a negative displacement suggests movement in the opposite direction, in this case, leftward.
The sign of displacement is of immense importance in real-world applications. Consider a scenario where a car travels 50 kilometers in the westward direction. The displacement of the car would be -50 kilometers. The negative sign here explicitly conveys that the car has moved to the left of its initial position.
This directional information is critical in various fields. In navigation, for example, pilots and sailors rely heavily on the sign of displacement to determine their exact location. By carefully tracking the changes in their position, they can ensure they are heading in the intended direction and avoid getting lost.
In conclusion, the sign of displacement plays a vital role in understanding the direction of movement of an object. It serves as a guiding force in navigation, engineering, and countless other disciplines, allowing us to accurately determine the trajectory of objects and plan our movements accordingly.
Velocity Function: Derivative of the Position Function
In our quest to unravel the mysteries of displacement, let us delve into the concept of a velocity function and its intricate connection with the position function. Understanding this relationship is crucial for mastering the art of finding displacement using the weapons of calculus.
The velocity function, symbolized as v(t), holds the key to unlocking the rate of change of an object’s position over time. It is defined as the derivative of the position function, s(t), which represents the object’s position at time t. In mathematical terms, this relationship is expressed as v(t) = ds(t)/dt.
Just as the velocity function measures the rate of change of position, it is itself subject to another layer of change, governed by acceleration. Acceleration, denoted as a(t), measures the rate at which velocity changes over time. This intricate web of relationships is captured by the second derivative of the position function, which yields the acceleration function: a(t) = dv(t)/dt.
Therefore, we can unravel the secrets of displacement by skillfully wielding the following formula:
Displacement = ∫v(t)dt
This formula serves as a powerful tool, allowing us to determine the net change in an object’s position over a given time interval by integrating its velocity function.
In essence, the velocity function unveils the dynamic nature of an object’s movement, revealing its speed and direction at any given instant. By mastering the relationship between velocity, acceleration, and position, we empower ourselves to conquer the challenges of displacement calculations, illuminating the path to understanding the intricate dance of objects in motion.