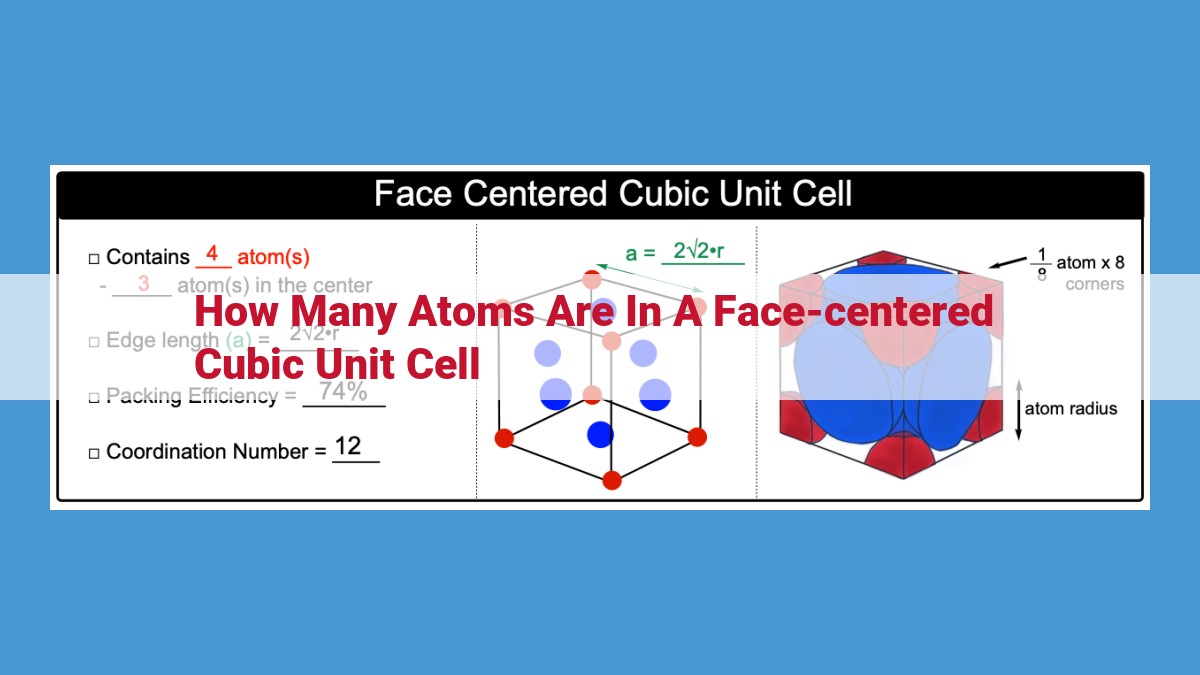
4 atoms. A face-centered cubic unit cell has 8 lattice points at the corners and 6 face-centered atoms at the centers of faces. Each corner atom is shared by 8 unit cells, while each face-centered atom is shared by 2 unit cells. The stoichiometry of the unit cell is ABCABC, so each unit cell contains 4 atoms.
Understanding Face-Centered Cubic Unit Cell
In the realm of materials science, the arrangement of atoms and molecules within a material’s structure plays a pivotal role in determining its properties. One fundamental way to describe this arrangement is through the concept of unit cells. A unit cell is the smallest repeating unit of a crystal lattice that contains all the information about the crystal structure.
Among the various types of unit cells, the face-centered cubic (FCC) unit cell stands out. It is characterized by its compact and highly symmetrical arrangement of atoms. Imagine a cube with atoms located at each corner and in the center of each of the six faces. This arrangement creates a highly efficient packing of atoms, granting FCC structures a high density.
The lattice points of an FCC unit cell refer to the specific locations within the cube where atoms reside. These points form the framework of the crystal lattice and determine the overall symmetry of the structure. Each atomic position within the FCC unit cell is equidistant from its nearest neighbor atoms, contributing to the crystal’s stability.
The basis of a unit cell refers to the number of atoms it contains. In an FCC unit cell, the basis is 4, representing the four atoms located at the corners and the center of each face of the cube. This basis value plays a crucial role in determining the stoichiometry and properties of the crystal.
The atomic packing efficiency (APE) of a crystal structure measures how efficiently atoms are packed within the unit cell. FCC structures are known for their high APE, with a value of 74%. This high efficiency results from the compact arrangement of atoms, which minimizes wasted space within the crystal.
In summary, the face-centered cubic unit cell is a fundamental building block in materials science. Its compact arrangement of atoms, high symmetry, and efficient packing contribute to the distinct properties and applications of FCC materials. Understanding the intricacies of this unit cell provides valuable insights into the atomic-level structure and behavior of materials.
Lattice Points and Atomic Positions in a Face-Centered Cubic Unit Cell
In the world of crystal structures, the face-centered cubic (FCC) unit cell stands out as a fascinating and highly symmetrical arrangement of atoms. To truly understand this structure, we must delve into the realm of lattice points and atomic positions.
Lattice Points: The Foundation of FCC
Lattice points serve as the backbone of a crystal structure, defining the regular arrangement of atoms. In an FCC unit cell, there are eight lattice points positioned at each corner of the cube and six lattice points centered on each face. These lattice points represent the locations where atoms can reside.
Atomic Positions: Where Atoms Dwell
The atomic positions in an FCC unit cell are anything but random. Atoms occupy lattice points, but not all lattice points are created equal. The corner lattice points are shared by eight adjacent unit cells, while the face-centered lattice points are shared by two adjacent unit cells.
Due to the close proximity of atoms in an FCC structure, each atom is surrounded by 12 nearest neighbors, forming a compact and efficient arrangement. This close-packing is responsible for the high density and strength of FCC materials.
The Significance of Lattice Points and Atomic Positions
The arrangement of lattice points and atomic positions in an FCC unit cell has profound implications for the properties of materials. For instance, the high symmetry of the FCC structure allows for multiple slip planes, making FCC materials highly ductile.
Additionally, the close-packing of atoms minimizes interstitial voids, reducing the likelihood of defects and enhancing the material’s mechanical strength.
Understanding the lattice points and atomic positions in an FCC unit cell is crucial for comprehending the behavior, properties, and applications of FCC materials in various fields.
The Basis of a Face-Centered Cubic Unit Cell
A face-centered cubic (FCC) unit cell is a three-dimensional structure that forms the foundation of many metallic crystals. It consists of cubic shape with atoms positioned at each corner and in the center of each face, giving it a total of 14 atoms per unit cell.
The basis of a unit cell refers to the number of atoms it contains. In the case of FCC unit cells, the basis is 4. This means that the unit cell can be thought of as containing 4 atoms, which repeat themselves throughout the crystal structure.
Atomic Packing Efficiency
Atomic packing efficiency measures how efficiently atoms are packed together within a unit cell. It is calculated by dividing the volume occupied by atoms by the total volume of the unit cell. The higher the packing efficiency, the more closely the atoms are packed.
FCC unit cells have a very high atomic packing efficiency of 74%. This means that the atoms are packed together very efficiently, leaving little empty space between them. This high packing efficiency contributes to the strength and stability of FCC crystal structures.
In contrast, other types of unit cells, such as body-centered cubic (BCC) and hexagonal close-packed (HCP), have lower packing efficiencies of 68% and 74%, respectively. This difference in packing efficiency can affect the properties of materials with different crystal structures.
Calculating the Volume of a Face-Centered Cubic Unit Cell
In the realm of crystallography, understanding the structure of materials is crucial for deciphering their properties and behavior. One of the key parameters in this exploration is the volume of the unit cell, the smallest repeating unit that characterizes a crystal. For a face-centered cubic (FCC) unit cell, a specific mathematical formula guides us in determining its volume, unraveling essential insights into the crystal’s atomic arrangement.
To embark on this calculation, we must first grasp the concept of the lattice parameter, denoted as a. It represents the length of the unit cell’s edge, which is the distance between adjacent lattice points. These lattice points, like invisible scaffolding, define the framework upon which the atoms reside.
In an FCC unit cell, the atoms are not only positioned at the corners of the cube but also at the centers of each face. This unique arrangement gives the FCC structure a high packing efficiency, meaning that the atoms are packed together as densely as possible.
With this foundation, we can delve into the formula for calculating the volume of an FCC unit cell:
**Volume = a^3**
Here, a is the lattice parameter. This simple equation eloquently expresses the volume of the unit cell as the cube of its edge length. By plugging in the appropriate value for a, we can swiftly determine the volume of the unit cell.
Comprehending the volume of an FCC unit cell is paramount for understanding the material’s properties. A larger volume implies a less dense packing of atoms, while a smaller volume indicates a denser arrangement. This knowledge empowers materials scientists and engineers to tailor materials with specific properties for diverse applications.
Determining the Number of Atoms in a Face-Centered Cubic Unit Cell
- Explain the stoichiometry and basis of a face-centered cubic unit cell.
- Calculate the number of atoms in a face-centered cubic unit cell based on its structure.
Determining the Number of Atoms in a Face-Centered Cubic Unit Cell
In the realm of materials science, understanding the arrangement of atoms within a crystal structure is crucial. Among the different crystal structures, the face-centered cubic (FCC) unit cell stands out for its unique atomic arrangement and practical applications.
Stoichiometry and Basis of an FCC Unit Cell
An FCC unit cell consists of 14 lattice points, which are points that define the location of atoms in the crystal. Of these, 8 lattice points lie at the corners of the cube, while 6 occupy the center of each face. The basis of an FCC unit cell, which represents the number of atoms associated with each lattice point, is 4.
Calculating the Number of Atoms
To determine the total number of atoms in an FCC unit cell, we multiply the number of lattice points by the basis. Since there are 14 lattice points and a basis of 4, the FCC unit cell contains 56 atoms.
Arrangement of Atoms in an FCC Unit Cell
The atoms in an FCC unit cell are arranged in a highly efficient manner. The 14 lattice points are surrounded by 12 neighboring atoms, while each corner atom is adjacent to 8 others. This arrangement maximizes the packing efficiency of the unit cell, resulting in a high density of atoms compared to other crystal structures.
Applications of FCC Structures
FCC structures are widely encountered in various materials with exceptional properties. Aluminum, copper, gold, silver, and lead are notable examples of metals with FCC crystal structures. These materials exhibit high strength, ductility, and electrical and thermal conductivity.
Determining the number of atoms in a face-centered cubic unit cell not only provides insights into the atomic arrangement but also highlights the unique characteristics and applications of this crystal structure. The efficient packing of atoms in FCC unit cells results in desirable material properties and contributes to their widespread use in engineering and technology.
Summary and Applications of Face-Centered Cubic Unit Cells
To summarize, a face-centered cubic (FCC) unit cell, characterized by its cubic shape with atoms at each corner and the center of each face, contains 4 atoms. This unique arrangement results in an atomic packing efficiency of 74%, making FCC unit cells particularly dense and stable.
The FCC structure is highly relevant in materials science and engineering due to its exceptional properties. Materials with FCC structures often exhibit:
- High strength and hardness due to the close packing of atoms, making them suitable for applications such as steel production.
- Ductility due to the ability of atoms to slide past each other without breaking bonds, enhancing malleability and ductility.
- Thermal conductivity due to the efficient transfer of heat through the tightly packed atomic structure.
Examples of FCC materials include:
- Metals: Aluminum, copper, nickel, and iron
- Alloys: Stainless steel, brass, and bronze
- Minerals: Diamond, zinc blende, and pyrite
The FCC unit cell provides a fundamental understanding of the atomic arrangements and properties of these materials, enabling scientists and engineers to design and optimize their performance for a wide range of applications, from structural components to electronic devices.