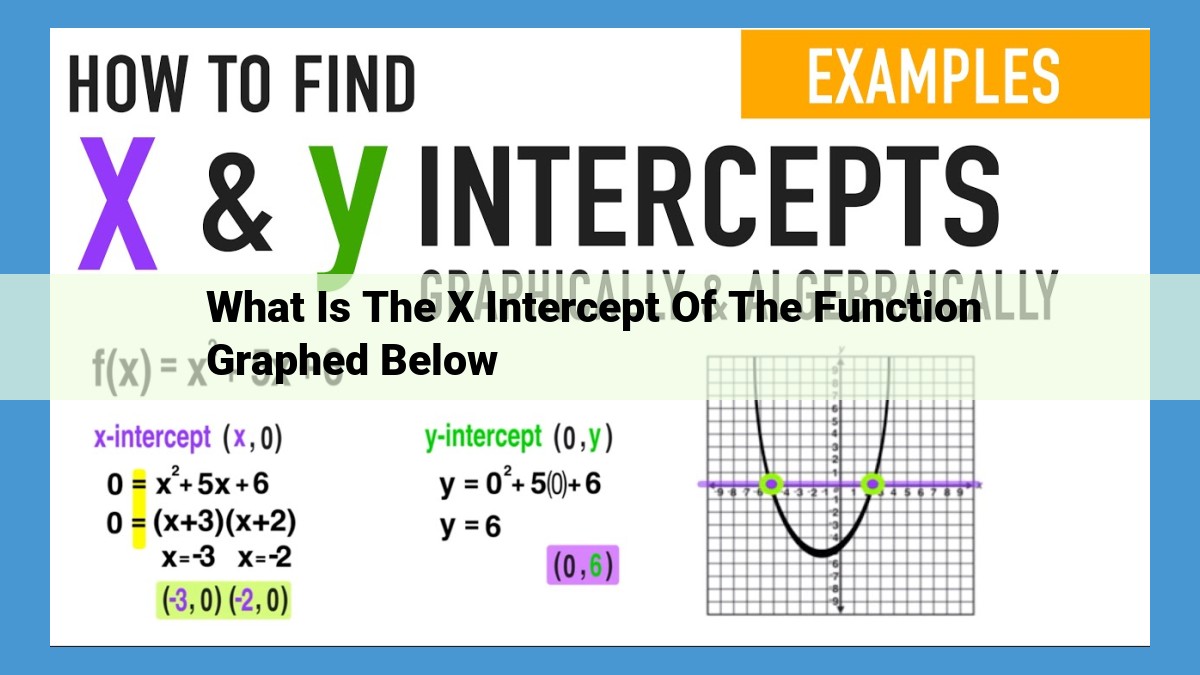
An x-intercept is a point where a function’s graph crosses the x-axis, indicating that the function’s value is zero at that point. The x-intercept is found by determining the value of x where the function’s equation equals zero. It represents the point where the graph intersects the horizontal axis, providing insight into the function’s behavior and properties. Understanding x-intercepts is crucial for analyzing linear and non-linear functions and their relationship to the coordinate plane.
Understanding Intercepts: The Essence of Graphing Linear Equations
When visualizing linear equations on a graph, intercepts play a pivotal role in defining the line’s position and behavior. They represent the points where the line crosses the y-axis (vertical axis) and the x-axis (horizontal axis).
The y-intercept is the point where the line intersects the y-axis. It indicates the value of the dependent variable (usually represented by y) when the independent variable (usually represented by x) is equal to zero. In other words, it tells us the starting point of the line along the vertical axis.
In contrast, the x-intercept is the point where the line intersects the x-axis. It indicates the value of the independent variable when the dependent variable is equal to zero. This point reveals where the line crosses the horizontal axis, providing insight into the line’s behavior.
Understanding intercepts is crucial for interpreting and analyzing linear equations. They offer valuable information about the equation’s origin, direction, and relationship with the coordinate axes.
The Mathematics of X-Coordinates
- Significance of x-coordinates in describing a point’s location
- Relationship between x-coordinates and the abscissa
The Mathematics of X-Coordinates: A Guide to Mapping Points
In the realm of mathematics, coordinates play a crucial role in defining the exact location of points within a plane. Among these coordinates, x-coordinates hold immense significance in describing a point’s position and understanding the relationship between its location and the overall structure.
X-Coordinates: The Horizontal Locator
Imagine a plane divided into four quadrants by two perpendicular lines known as axes. The x-axis is a horizontal line that runs from left to right, while the y-axis runs vertically from bottom to top. Together, they create a coordinate grid that allows us to pinpoint any point within the plane.
The x-coordinate of a point represents its horizontal displacement from the origin (the point where the axes intersect). It tells us how far the point is located to the right or left of the y-axis. Positive x-coordinates indicate a point to the right of the y-axis, while negative x-coordinates indicate a point to the left.
Relationship with the Abscissa
The abscissa is simply the x-coordinate of a point. It is often used interchangeably with the term “x-coordinate” and plays a vital role in various mathematical concepts such as graphing, distance calculations, and transformations.
By understanding the significance of x-coordinates and their relationship with the abscissa, we gain a powerful tool for defining and manipulating points within a plane. This understanding forms the foundation for further exploration of geometry, trigonometry, and other mathematical fields that rely heavily on the precise location and movement of points.
The Concept of Axes: Dividing the Plane
In the realm of geometry, the concept of axes plays a pivotal role in structuring and defining a plane. These axes, like invisible rulers, intersect at a single point, creating a coordinate system that helps us pinpoint the location of points and understand the behavior of lines and other geometric objects.
The most fundamental axes are the x-axis and the y-axis. The x-axis extends horizontally, while the y-axis runs vertically, forming a Cartesian plane. This plane is divided into four quadrants by the axes, each with its own unique combination of positive and negative signs.
The x-axis is often referred to as the abscissa, while the y-axis is called the ordinate. These terms are used to describe the coordinates of a point, which specify its position relative to the axes. The x-coordinate, represented by the letter x, indicates the point’s distance from the y-axis, while the y-coordinate, represented by the letter y, indicates its distance from the x-axis.
The intersection of the x- and y-axes creates the origin, a special point where both coordinates are zero. This point serves as the reference point for all other points in the plane, allowing us to determine their relative positions.
By understanding the role of axes in dividing a plane and identifying the x-axis and y-axis, we gain a foundational understanding of Cartesian geometry. This knowledge empowers us to locate points, describe lines, and solve geometric problems with greater precision and ease.
Delving into the World of Graphs: A Beginner’s Guide to Visualizing Data
Graphs are a fundamental tool for understanding and interpreting data. They provide a visual representation that allows us to see patterns, trends, and relationships that may not be readily apparent from the raw data.
Definition and Purpose of Graphs:
A graph is a diagram that shows the relationship between two or more variables. The horizontal axis (also known as the x-axis) represents the independent variable, while the vertical axis (or y-axis) represents the dependent variable. Each point on the graph represents a specific combination of the two variables.
Understanding Plotting and Charts:
Plotting involves placing points on the graph according to their respective values. These points are then connected to form lines, curves, or other shapes. The resulting diagram is known as a chart. Charts can be used to visualize various types of data, such as bar charts, line charts, and scatter plots.
Graphs empower us to visualize and interpret data in a way that makes it easier to understand complex relationships and draw meaningful conclusions. By understanding the concepts of plotting and charts, we gain the ability to effectively represent and communicate data for a wide range of purposes.
Properties of Lines
- Definition and characteristics of a line
- Description of line segments
Properties of Lines: Unveiling the Essence of Lines
In the realm of geometry, lines play a pivotal role, defining the shape and structure of various figures. They possess distinct characteristics that distinguish them from other geometric entities, making them indispensable in the world of mathematics.
A line can be defined as a straight path extending infinitely in both directions. It has no thickness or width, existing only as a one-dimensional object. Lines possess several key attributes that help us understand their behavior and properties.
One defining feature of a line is its length. Unlike line segments, which have a finite length and are bounded by endpoints, lines extend endlessly. This continuous nature allows lines to stretch indefinitely, creating an open and unbounded path.
Line Segments: The Building Blocks of Lines
While lines stretch infinitely, line segments represent finite portions of lines. They are characterized by endpoints that mark their beginnings and ends. Line segments can be of varying lengths, and they play a crucial role in constructing more complex geometric shapes, such as triangles and quadrilaterals.
The length of a line segment is measured by the distance between its endpoints. This distance can be calculated using the distance formula, which involves the difference between the x-coordinates and y-coordinates of the endpoints.
In summary, lines and line segments are fundamental geometric entities with unique properties. Understanding their characteristics is essential for navigating the world of geometry and appreciating the fascinating relationships that exist within it.
Intersection Points: Unraveling the Hidden Interplay of Lines
In the realm of geometry, intersection points hold immense significance, acting as gateways where two or more lines cross paths. These pivotal points reveal a captivating dance between lines, offering valuable insights into their behavior and characteristics.
Determining the Coordinates of an Intersection
Unveiling the secrets of an intersection point requires a precise understanding of its x-coordinate and y-coordinate. The x-coordinate pinpoints its location along the x-axis, while the y-coordinate does the same on the y-axis. Together, these coordinates provide an exact address for the intersection point on the coordinate plane.
To determine the coordinates, we embark on a systematic process. First, we solve the equations of the lines simultaneously to find the values of x and y that satisfy both equations. These values represent the x-coordinate and y-coordinate of the intersection point.
The Value of Intersection Points
Intersection points are not mere abstract concepts; they carry practical significance. In various fields, including physics, engineering, and computer graphics, the ability to determine intersection points is crucial for solving complex problems. For instance, in architectural design, finding the intersection point of two walls helps determine their angle of intersection.
By mastering the art of finding intersection points, we gain a deeper understanding of the relationships between lines and their behavior on the coordinate plane. These insights empower us to analyze and predict the outcomes of geometrical scenarios, unlocking new possibilities for problem-solving and exploration.
Understanding Points in a Plane: The Foundation of Coordinate Geometry
In the realm of mathematics, points hold a pivotal role in defining the intricate tapestry of geometric shapes and figures. Understanding the nature and characteristics of points is paramount for navigating the landscape of coordinate geometry, where points become the building blocks of our understanding of shapes and their relationships.
A point, in its essence, is a fundamental geometric object that lacks length, width, or height. It represents a precise location in a plane, akin to a tiny dot on a map. Points are often represented symbolically using capital letters, such as P, Q, or R.
When working with points, their coordinates become essential for describing their precise position within the Cartesian coordinate system. The Cartesian coordinate system is an ingenious system that divides a plane into four quadrants using two perpendicular axes: the x-axis and the y-axis.
To specify a point in a plane, we use ordered pairs of numbers, known as coordinates. The first number in the ordered pair represents the point’s distance from the y-axis, while the second number represents its distance from the x-axis. By combining these two numbers, we pinpoint the exact location of the point.
For instance, the point (3, 4) lies 3 units to the right of the y-axis and 4 units above the x-axis. This precise coordinate notation allows us to locate points with accuracy and clarity.
Points play a crucial role in various mathematical operations and concepts. They serve as the vertices of polygons, the endpoints of line segments, and the centers of circles. By understanding the nature and characteristics of points, we gain a solid foundation for understanding the broader world of geometry.
The Concept of X-Intercepts: Unraveling Its Significance
In the realm of mathematics, understanding the concept of x-intercepts is crucial for navigating the world of graphs and equations. An x-intercept represents a unique point where a graph crosses the x-axis. This point holds significant value in describing the behavior of lines and equations.
Definition of X-Intercept
An x-intercept is defined as the point where a line or curve intersects the x-axis. At this point, the y-coordinate of the graph is zero. In other words, it indicates the value of x when y equals 0.
Relationship with Y-Intercepts
X-intercepts and y-intercepts are closely related. The y-intercept represents the point where a graph crosses the y-axis, where the x-coordinate is zero. Together, x-intercepts and y-intercepts provide valuable information about the line’s position and characteristics.
Importance in Understanding Line Behavior
X-intercepts play a vital role in understanding the behavior of lines. They can reveal:
- Zeroes of a function: If a function is equated to zero, the x-intercepts represent the zeroes of the function. These values indicate where the function equals zero.
- Symmetry: If a line has two x-intercepts that are equal in magnitude but opposite in sign, it exhibits symmetry about the origin.
- Domain and range: X-intercepts help determine the domain and range of a function. The domain represents the set of all possible x-values, while the range represents the set of all possible y-values.
By understanding the concept of x-intercepts, you gain a deeper comprehension of graphs, equations, and the behavior of lines. They serve as valuable tools for solving problems, analyzing data, and making informed decisions.